


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 16-2-2016
Date: 23-11-2021
Date: 25-9-2021
|

A totalistic cellular automaton is a cellular automata in which the rules depend only on the total (or equivalently, the average) of the values of the cells in a neighborhood. These automata were introduced by Wolfram in 1983. Like an elementary cellular automaton, the evolution of a one-dimensional totalistic cellular automaton can completely be described by a table specifying the state a given cell will have in the next generation based on the average value of the three cells consisting of the cell to its left, the value the cell itself, and the value of the cell to its right.
For a 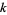 -color one-dimensional totalistic automaton, there are
-color one-dimensional totalistic automaton, there are 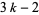 possible states for the average of three cells neighboring a given cell, and a total of
possible states for the average of three cells neighboring a given cell, and a total of 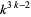
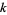 -color totalistic cellular automata, each of which can be indexed with an
-color totalistic cellular automata, each of which can be indexed with an 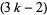 -digit
-digit 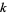 -ary number, known as a "code." For example, the table giving the evolution of the 3-color code
-ary number, known as a "code." For example, the table giving the evolution of the 3-color code  is illustrated above. In this diagram, the possible average values of the three neighboring cells are shown in the top row of each panel, and the resulting value the central cell takes in the next generation is shown below in the center.
is illustrated above. In this diagram, the possible average values of the three neighboring cells are shown in the top row of each panel, and the resulting value the central cell takes in the next generation is shown below in the center. 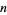 generations of the totalistic cellular automaton code
generations of the totalistic cellular automaton code 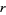 with
with 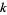 colors is implemented in as CellularAutomaton[
colors is implemented in as CellularAutomaton[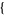 r,
r, 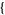 3, 1
3, 1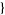
 ,
, 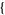
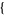 1
1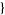 , 0
, 0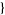 , n,
, n, 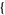 All, All
All, All ].
].

The evolution of a one-dimensional cellular automaton can be illustrated by starting with the initial state (generation zero) in the first row, the first generation on the second row, and so on. For example, the figures above illustrate the first 20 generations of the code 777 3-color totalistic cellular automaton starting with a single gray cell (left figure) and a single black cell (right figure).

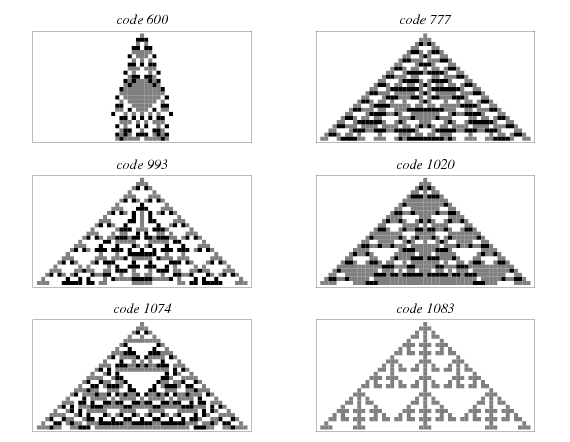
The illustrations above show automata numbers 600, 777, 993, 1020, 1074, and 1083 propagated for 25 generations.
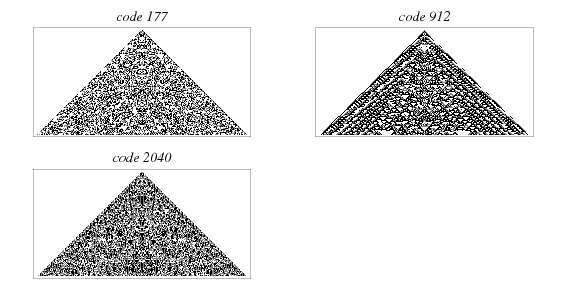
The illustrations above show one-dimensional automata that display apparently random features.
The best known two-dimensional totalistic cellular automaton is the game of life.
REFERENCES:
Rangel-Mondragon, J. "A Catalog of Cellular Automata." http://library.wolfram.com/infocenter/MathSource/505/.
Wolfram, S. "Statistical Mechanics of Cellular Automata." Rev. Mod. Phys. 55, 601-644, 1983.
Wolfram, S. "Universality and Complexity in Cellular Automata." Physica D 10, 1-35, 1984.
Wolfram, S. "Twenty Problems in the Theory of Cellular Automata." Physica Scripta T9, 170-183, 1985.
Wolfram, S. A New Kind of Science. Champaign, IL: Wolfram Media, pp. 60-70 and 886, 2002.



|
|
|
|
للعاملين في الليل.. حيلة صحية تجنبكم خطر هذا النوع من العمل
|
|
|
|
|
|
|
"ناسا" تحتفي برائد الفضاء السوفياتي يوري غاغارين
|
|
|
|
|
|
|
نحو شراكة وطنية متكاملة.. الأمين العام للعتبة الحسينية يبحث مع وكيل وزارة الخارجية آفاق التعاون المؤسسي
|
|
|