

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Klein Bottle
المؤلف:
Apéry, F
المصدر:
Models of the Real Projective Plane. Braunschweig, Germany: Vieweg, 1987.
الجزء والصفحة:
...
12-8-2021
4995
Klein Bottle
 |
 |
The Klein bottle is a closed nonorientable surface of Euler characteristic 0 (Dodson and Parker 1997, p. 125) that has no inside or outside, originally described by Felix Klein (Hilbert and Cohn-Vossen 1999, p. 308). It can be constructed by gluing both pairs of opposite edges of a rectangle together giving one pair a half-twist, but can be physically realized only in four dimensions, since it must pass through itself without the presence of a hole. Its topology is equivalent to a pair of cross-caps with coinciding boundaries (Francis and Weeks 1999). It can be represented by connecting the side of a square in the orientations illustrated in the right figure above (Gardner 1984, pp. 15-17; Gray 1997, pp. 323-324).
It can be cut in half along its length to make two Möbius strips (Dodson and Parker 1997, p. 88), but can also be cut into a single Möbius strip (Gardner 1984, pp. 14 and 17).
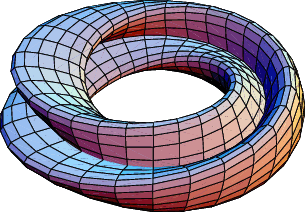
The above picture is an immersion of the Klein bottle in 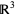 (three-space). There is also another possible immersion called the "figure-8" immersion (Apéry 1987, Gray 1997, Geometry Center). While Gray (1997) depicts this embedding using the eight curve (aka. lemniscate of Gerono), it can also be constructed using the usual (Bernoulli) lemniscate (Pinkall 1985, Apéry 1987).
(three-space). There is also another possible immersion called the "figure-8" immersion (Apéry 1987, Gray 1997, Geometry Center). While Gray (1997) depicts this embedding using the eight curve (aka. lemniscate of Gerono), it can also be constructed using the usual (Bernoulli) lemniscate (Pinkall 1985, Apéry 1987).
The equation for the usual immersion is given by the implicit equation
![(x^2+y^2+z^2+2y-1)[(x^2+y^2+z^2-2y-1)^2-8z^2]
+16xz(x^2+y^2+z^2-2y-1)=0](https://mathworld.wolfram.com/images/equations/KleinBottle/NumberedEquation1.gif) |
(1) |
(Stewart 1991). Nordstrand gives the parametric form
 |
 |
![cosu[cos(1/2u)(sqrt(2)+cosv)+sin(1/2u)sinvcosv]](https://mathworld.wolfram.com/images/equations/KleinBottle/Inline4.gif) |
(2) |
 |
 |
![sinu[cos(1/2u)(sqrt(2)+cosv)+sin(1/2u)sinvcosv]](https://mathworld.wolfram.com/images/equations/KleinBottle/Inline7.gif) |
(3) |
 |
 |
 |
(4) |
The "figure-8" form of the Klein bottle is obtained by rotating a figure eight about an axis while placing a twist in it, and is given by parametric equations
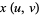 |
 |
![[a+cos(1/2u)sin(v)-sin(1/2u)sin(2v)]cos(u)](https://mathworld.wolfram.com/images/equations/KleinBottle/Inline13.gif) |
(5) |
 |
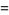 |
![[a+cos(1/2u)sin(v)-sin(1/2u)sin(2v)]sin(u)](https://mathworld.wolfram.com/images/equations/KleinBottle/Inline16.gif) |
(6) |
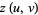 |
 |
 |
(7) |
for  ,
, 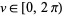 , and
, and 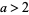 (Gray 1997).
(Gray 1997).
The image of the cross-cap map of a torus centered at the origin is a Klein bottle (Gray 1997, p. 339). The Möbius shorts are topologically equivalent to a Klein bottle with a hole (Gramain 1984, Stewart 2000).
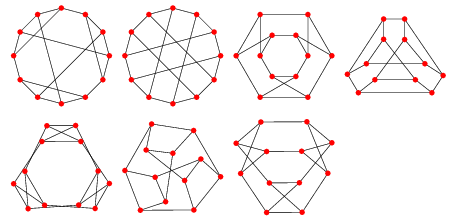

Any set of regions on the Klein bottle can be colored using six colors only (Franklin 1934, Saaty and Kainen 1986), providing the sole exception to the Heawood conjecture (Bondy and Murty 1976, p. 244). The 12-vertex graph (top figures) whose embedding on the Klein bottle (bottom figure) divides it into regions having a minimal coloring using six colors is known as the Franklin graph.
REFERENCES:
Apéry, F. Models of the Real Projective Plane. Braunschweig, Germany: Vieweg, 1987.
Bondy, J. A. and Murty, U. S. R. Graph Theory with Applications. New York: North Holland, p. 244, 1976.
Dickson, S. "Klein Bottle Graphic." http://library.wolfram.com/infocenter/MathSource/4560/.
Dodson, C. T. J. and Parker, P. E. A User's Guide to Algebraic Topology. Dordrecht, Netherlands: Kluwer, 1997.
Francis, G. K. and Weeks, J. R. "Conway's ZIP Proof." Amer. Math. Monthly 106, 393-399, 1999.
Franklin, P. "A Six Colour Problem." J. Math. Phys. 13, 363-369, 1934.
Gardner, M. "Klein Bottles and Other Surfaces." Ch. 2 in The Sixth Book of Mathematical Games from Scientific American. Chicago, IL: University of Chicago Press, pp. 9-18, 1984.
Geometry Center. "The Klein Bottle." http://www.geom.umn.edu/zoo/toptype/klein/.
Geometry Center. "The Klein Bottle in Four-Space." http://www.geom.umn.edu/~banchoff/Klein4D/Klein4D.html.
Gramain, A. Topology of Surfaces. Moscow, ID: BCS Associates, 1984.
Gray, A. "The Klein Bottle" and "A Different Klein Bottle." §14.4 and 14.5 in Modern Differential Geometry of Curves and Surfaces with Mathematica, 2nd ed. Boca Raton, FL: CRC Press, pp. 327-330, 1997.
Hilbert, D. and Cohn-Vossen, S. Geometry and the Imagination. New York: Chelsea, pp. 308-311, 1999.
JavaView. "Classic Surfaces from Differential Geometry: Klein Bottle." http://www-sfb288.math.tu-berlin.de/vgp/javaview/demo/surface/common/PaSurface_KleinBottle.html.
Nordstrand, T. "The Famed Klein Bottle." http://jalape.no/math/kleintxt.
Pappas, T. "The Moebius Strip & the Klein Bottle." The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, pp. 44-46, 1989.
Pinkall, U. "Regular Homotopy Classes of Immersed Surfaces." Topology 24, 421-434, 1985.
Saaty, T. L. and Kainen, P. C. The Four-Color Problem: Assaults and Conquest. New York: Dover, p. 45, 1986.
Stewart, I. Game, Set and Math. New York: Viking Penguin, 1991.
Stewart, I. "Mathematical Recreations: Reader Feedback." Sci. Amer. 283, 101, Sep. 2000.
Stoll, C. "Acme Klein Bottle." http://www.kleinbottle.com/.
Trott, M. "Constructing an Algebraic Klein Bottle." Mathematica in Educ. Res. 8, 24-27, 1999. http://library.wolfram.com/infocenter/Articles/2077/.
Trott, M. "The Mathematica Guidebooks Additional Material: Klein Bottle with Hexagonal Wireframe." http://www.mathematicaguidebooks.org/additions.shtml#G_2_03.
Underwood, M. "Mobius Scarf, Klein Bottle, Klein Bottle 'Hat'." http://www.woolworks.org/patterns/klein.txt.
Wang, P. "Renderings." http://www.ugcs.caltech.edu/~peterw/portfolio/renderings/ Wells, D. The Penguin Dictionary of Curious and Interesting Geometry. London: Penguin, pp. 131-132, 1991.
Wolfram Research, Inc. "Algebraic Construction of a Klein Bottle." http://library.wolfram.com/infocenter/Demos/119/.
 الاكثر قراءة في التبلوجيا
الاكثر قراءة في التبلوجيا
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















