

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Knot Symmetry
المؤلف:
Bonahon, F. and Siebermann, L.
المصدر:
"The Classification of Algebraic Links." Unpublished manuscript.
الجزء والصفحة:
...
19-6-2021
1674
Knot Symmetry
A symmetry of a knot 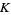 is a homeomorphism of
is a homeomorphism of  which maps
which maps  onto itself. More succinctly, a knot symmetry is a homeomorphism of the pair of spaces
onto itself. More succinctly, a knot symmetry is a homeomorphism of the pair of spaces 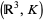 . Hoste et al. (1998) consider four types of symmetry based on whether the symmetry preserves or reverses orienting of
. Hoste et al. (1998) consider four types of symmetry based on whether the symmetry preserves or reverses orienting of 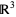 and
and 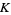 ,
,
1. preserves 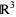 , preserves
, preserves 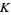 (identity operation),
(identity operation),
2. preserves 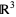 , reverses
, reverses 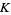 ,
,
3. reverses  , preserves
, preserves 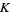 ,
,
4. reverses 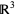 , reverses
, reverses 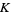 .
.
This then gives the five possible classes of symmetry summarized in the table below.
| class | symmetries | knot symmetries |
 |
1 | chiral, noninvertible |
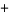 |
1, 3 | 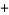 amphichiral, noninvertible amphichiral, noninvertible |
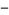 |
1, 4 | 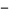 amphichiral, noninvertible amphichiral, noninvertible |
 |
1, 2 | chiral, invertible |
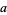 |
1, 2, 3, 4 |  and and  amphichiral, invertible amphichiral, invertible |
In the case of hyperbolic knots, the symmetry group must be finite and either cyclic or dihedral (Riley 1979, Kodama and Sakuma 1992, Hoste et al. 1998). The classification is slightly more complicated for nonhyperbolic knots. Furthermore, all knots with 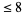 crossings are either amphichiral or invertible (Hoste et al. 1998). Any symmetry of a prime alternating link must be visible up to flypes in any alternating diagram of the link (Bonahon and Siebermann, Menasco and Thistlethwaite 1993, Hoste et al. 1998).
crossings are either amphichiral or invertible (Hoste et al. 1998). Any symmetry of a prime alternating link must be visible up to flypes in any alternating diagram of the link (Bonahon and Siebermann, Menasco and Thistlethwaite 1993, Hoste et al. 1998).
 |
 |
The following tables (Hoste et al. 1998) give the numbers of 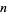 -crossing knots belonging to cyclic symmetry groups
-crossing knots belonging to cyclic symmetry groups 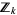 (Sloane's A052411 for
(Sloane's A052411 for 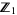 and A052412 for
and A052412 for  ) and dihedral symmetry groups
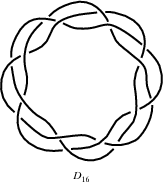
) and dihedral symmetry groups 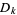 (Sloane's A052415 through A052422). Of knots with 16 or fewer crossings, there are only one each having symmetry groups
(Sloane's A052415 through A052422). Of knots with 16 or fewer crossings, there are only one each having symmetry groups 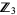 ,
, 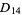 , and
, and 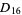 (above left). There are only two knots with symmetry group
(above left). There are only two knots with symmetry group 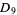 , one hyperbolic (above right), and one a satellite knot. In addition, there are 2, 4, and 10 satellite knots having 14-, 15-, and 16-crossings, respectively, which belong to the dihedral group
, one hyperbolic (above right), and one a satellite knot. In addition, there are 2, 4, and 10 satellite knots having 14-, 15-, and 16-crossings, respectively, which belong to the dihedral group 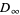 .
.
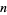 |
 |
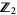 |
 |
 |
| 1 | 0 | 0 | 0 | 0 |
| 2 | 0 | 0 | 0 | 0 |
| 3 | 0 | 0 | 0 | 0 |
| 4 | 0 | 0 | 0 | 0 |
| 5 | 0 | 0 | 0 | 0 |
| 6 | 0 | 0 | 0 | 0 |
| 7 | 0 | 0 | 0 | 0 |
| 8 | 0 | 0 | 0 | 0 |
| 9 | 2 | 0 | 0 | 0 |
| 10 | 24 | 3 | 0 | 0 |
| 11 | 173 | 14 | 0 | 0 |
| 12 | 1047 | 57 | 0 | 0 |
| 13 | 6709 | 210 | 0 | 0 |
| 14 | 37177 | 712 | 0 | 2 |
| 15 | 224311 | 2268 | 1 | 0 |
| 16 | 1301492 | 7011 | 0 | 11 |
 |
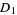 |
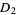 |
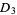 |
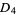 |
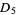 |
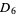 |
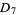 |
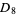 |
 |
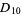 |
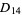 |
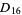 |
| 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 2 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 3 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 4 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 5 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 6 | 0 | 2 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 7 | 0 | 4 | 0 | 2 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 8 | 4 | 12 | 0 | 3 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 |
| 9 | 13 | 23 | 3 | 4 | 0 | 3 | 0 | 0 | 0 | 0 | 0 | 0 |
| 10 | 66 | 62 | 1 | 5 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 |
| 11 | 217 | 134 | 2 | 11 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 12 | 728 | 309 | 6 | 18 | 0 | 8 | 1 | 2 | 0 | 0 | 0 | 0 |
| 13 | 2391 | 647 | 1 | 21 | 2 | 3 | 1 | 2 | 0 | 0 | 0 | 0 |
| 14 | 7575 | 1463 | 4 | 31 | 2 | 2 | 0 | 0 | 0 | 0 | 1 | 0 |
| 15 | 23517 | 3065 | 50 | 53 | 3 | 12 | 0 | 2 | 1 | 4 | 0 | 0 |
| 16 | 73263 | 6791 | 15 | 89 | 0 | 10 | 1 | 8 | 1 | 1 | 0 | 1 |
REFERENCES:
Bonahon, F. and Siebermann, L. "The Classification of Algebraic Links." Unpublished manuscript.
Hoste, J.; Thistlethwaite, M.; and Weeks, J. "The First 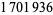 Knots." Math. Intell. 20, 33-48, Fall 1998.
Knots." Math. Intell. 20, 33-48, Fall 1998.
Kodama K. and Sakuma, M. "Symmetry Groups of Prime Knots Up to 10 Crossings." In Knot 90, Proceedings of the International Conference on Knot Theory and Related Topics, Osaka, Japan, 1990 (Ed. A. Kawauchi.) Berlin: de Gruyter, pp. 323-340, 1992.
Menasco, W. and Thistlethwaite, M. "The Classification of Alternating Links." Ann. Math. 138, 113-171, 1993.
Riley, R. "An Elliptic Path from Parabolic Representations to Hyperbolic Structures." In Topology of Low-Dimensional Manifolds, Proceedings, Sussex 1977 (Ed. R. Fenn). New York: Springer-Verlag, pp. 99-133, 1979.
Sloane, N. J. A. Sequences A052411, A052412, A052415, A052416, A052417, A052418, A052420, and A052422 in "The On-Line Encyclopedia of Integer Sequences."
 الاكثر قراءة في التبلوجيا
الاكثر قراءة في التبلوجيا
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















