Reidemeister Torsion
المؤلف:
Cheeger, J.
المصدر:
"Analytic Torsion and Reidemeister Torsion." Proc. Natl. Acad. Sci. USA 74
الجزء والصفحة:
...
17-5-2021
1491
Reidemeister Torsion
In algebraic topology, the Reidemeister torsion is a notion originally introduced as a topological invariant of 3-manifolds which has now been widely adapted to a variety of contexts. At the time of its discovery, the Reidemeister torsion was the first 3-manifold invariant able to distinguish between manifolds which are homotopy equivalent but not homeomorphic. Since then, the notion has been adapted to higher-dimensional manifolds, knots and links, dynamical systems, Witten's equations, and so on. In particular, it has a number of different definitions for various contexts.
For a commutative ring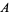 
, let 
be a finite acyclic chain complex of based finitely generated free R-modules of the form
 |
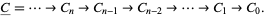(1)
|
The Reidemeister torsion of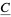 
is the value 
defined by
 |
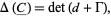(2)
|
where 
is the set of units of 
, 
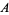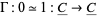is a chain contraction, 
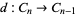is the boundary map, and
![d+Gamma=[d 0 0 ...; Gamma d 0 ...; 0 Gamma d ...; | | | ...]](https://mathworld.wolfram.com/images/equations/ReidemeisterTorsion/NumberedEquation3.gif) |
(3)
|
is a map from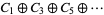 
to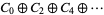 
. In this context, Reidemeister torsion is sometimes referred to as the torsion of the complex 
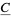(Nicolaescu 2002) and can be considered a generalization of the determinant of a matrix (Ranicki 1997).
Another common context for which to define Reidemeister torsion is in the case of CW-complexes. Begin with a compact metric space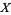 
with finite CW-decomposition 
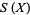and consider the canonically induced chain complex 
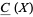of free Abelian groups,
 |
(4)
|
Lifting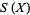 
to a CW-decomposition 
of the maximal Abelian cover 
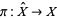of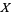 
yields an associated chain complex 
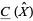which has a ![Z[H_1(X)]](https://mathworld.wolfram.com/images/equations/ReidemeisterTorsion/Inline20.gif)
basis. In particular, defining
 |
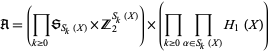(5)
|
where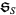 
denotes the group of permutations of a set 
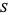, the torsion of the chain complex 
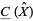of free ![Z[H_1(X)]](https://mathworld.wolfram.com/images/equations/ReidemeisterTorsion/Inline24.gif)
-modules with respect to the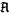 
-orbit of ![Z[H_1(X)]](https://mathworld.wolfram.com/images/equations/ReidemeisterTorsion/Inline26.gif)
-bases is called the Reidemeister torsion of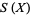 
. In this context, the Reidemeister torsion is a well-defined element of ![Q(Z[H_1(X)])/+/-H_1(X)](https://mathworld.wolfram.com/images/equations/ReidemeisterTorsion/Inline28.gif)
. In-depth details of this construction can be found in e.g., Nicolaescu (2002).
Reidemeister torsion is sometimes known as R-torsion or Reidemeister-Franz torsion. What's more, R-torsion is closely related to a number of other topological tools including Whitehead torsion, and was proven by Cheeger and Müller to be identically equal to the analytic torsion in the case of compact Riemannian manifolds.
SEE ALSO:Acyclic Chain Complex, Analytic Torsion, Basis, Chain, Chain Complex, Chain Contraction, Chain Homomorphism, Commutative Ring, Compact Manifold, Compact Space, Connected, Covering Space, CW-Complex, Determinant, Dynamical System, Free Abelian Group, Group, Group Generators, Group Orbit, Group Ring, Group Torsion, Homeomorphism, Homotopy Equivalence, Invariant, Knot, Link, Manifold, Metric Space, Module, Permutation Group, Quotient Group, R-Module, Riemannian Manifold, Torsion, Union, Unit, Unit Ring, Vector Basis, Whitehead Torsion, Witten's Equations
REFERENCES:
Cheeger, J. "Analytic Torsion and Reidemeister Torsion." Proc. Natl. Acad. Sci. USA 74, 2651-2654, 1977.
Nicolaescu, L. I. "Notes on the Reidemeister Torsion." 2002. https://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.8.4031&rep=rep1&type=pdf.
Ranicki, A. "Notes on Reidemeister Torsion." 1997. https://www.maths.ed.ac.uk/~aar/papers/torsion.pdf.
Turaev, V. G. "Reidemeister Torsion in Knot Theory." Uspekhi Mat. Nauk. 41, 97-147, 1986.
الاكثر قراءة في التبلوجيا
اخر الاخبار
اخبار العتبة العباسية المقدسة
