


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 27-10-2019
Date: 10-11-2020
Date: 11-11-2019
|
A set of 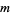 distinct positive integers
distinct positive integers  satisfies the Diophantus property
satisfies the Diophantus property 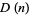 of order
of order 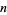 (a positive integer) if, for all
(a positive integer) if, for all 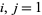 , ...,
, ...,  with
with  ,
,
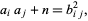 |
(1) |
the  s are integers. The set
s are integers. The set  is called a Diophantine
is called a Diophantine  -tuple.
-tuple.
Diophantine 1-doubles are abundant: (1, 3), (2, 4), (3, 5), (4, 6), (5, 7), (1, 8), (3, 8), (6, 8), (7, 9), (8, 10), (9, 11), ... (OEIS A050269 and A050270). Diophantine 1-triples are less abundant: (1, 3, 8), (2, 4, 12), (1, 8, 15), (3, 5, 16), (4, 6, 20), ... (OEIS A050273, A050274, and A050275).
Fermat found the smallest Diophantine 1-quadruple: 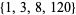 (Davenport and Baker 1969, Jones 1976). There are no others with largest term
(Davenport and Baker 1969, Jones 1976). There are no others with largest term  , and Davenport and Baker (1969) showed that if
, and Davenport and Baker (1969) showed that if 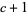 ,
, 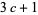 , and
, and 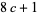 are all squares, then
are all squares, then 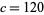 .
.
General 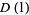 quadruples are
quadruples are
 |
(2) |
where 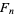 are Fibonacci numbers, and
are Fibonacci numbers, and
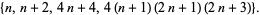 |
(3) |
The quadruplet
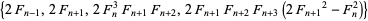 |
(4) |
is  (Dujella 1996). Dujella (1993) showed there exist no Diophantine quadruples
(Dujella 1996). Dujella (1993) showed there exist no Diophantine quadruples 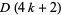 .
.
A longstanding conjecture is that no integer Diophantine quintuple exists (Gardner 1967, van Lint 1968, Davenport and Baker 1969, Kanagasabapathy and Ponnudurai 1975, Sansone 1976, Grinstead 1978).
Jones (1976) derived an infinite sequence of polynomials 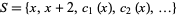 such that the product of any two consecutive polynomials, increased by 1, is the square of a polynomial. Letting
such that the product of any two consecutive polynomials, increased by 1, is the square of a polynomial. Letting 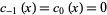 , then the general
, then the general 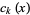 is given by the recurrence relation
is given by the recurrence relation
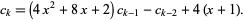 |
(5) |
The first few 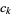 are
are
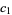 |
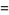 |
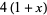 |
(6) |
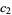 |
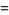 |
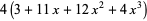 |
(7) |
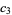 |
 |
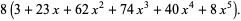 |
(8) |
Letting  gives the sequence
gives the sequence 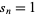 , 3, 8, 120, 1680, 23408, 326040, ... (OEIS A051047), for which
, 3, 8, 120, 1680, 23408, 326040, ... (OEIS A051047), for which 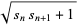 is 2, 5, 31, 449, 6271, 87361, ... (OEIS A051048).
is 2, 5, 31, 449, 6271, 87361, ... (OEIS A051048).
REFERENCES:
Brown, E. "Sets in Which 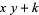 is Always a Square." Math. Comput. 45, 613-620, 1985.
is Always a Square." Math. Comput. 45, 613-620, 1985.
Davenport, H. and Baker, A. "The Equations 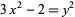 and
and  ." Quart. J. Math. (Oxford) Ser. 2 20, 129-137, 1969.
." Quart. J. Math. (Oxford) Ser. 2 20, 129-137, 1969.
Diofant Aleksandriĭskiĭ. Arifmetika i kniga o mnogougol'nyh chislakh [Russian]. Moscow: Nauka, 1974.
Dujella, A. "Generalization of a Problem of Diophantus." Acta Arith. 65, 15-27, 1993.
Dujella, A. "Diophantine Quadruples for Squares of Fibonacci and Lucas Numbers." Portugaliae Math. 52, 305-318, 1995.
Dujella, A. "Generalized Fibonacci Numbers and the Problem of Diophantus." Fib. Quart. 34, 164-175, 1996.
Dujella, A. "Diophantine 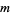 -Tuples-Introduction." https://web.math.hr/~duje/intro.html.
-Tuples-Introduction." https://web.math.hr/~duje/intro.html.
Gardner, M. "Mathematical Diversions." Sci. Amer. 216, 124, 1967.
Grinstead, C. M. "On a Method of Solving a Class of Diophantine Equations." Math. Comput. 32, 936-940, 1978.
Hoggatt, V. E. Jr. and Bergum, G. E. "A Problem of Fermat and the Fibonacci Sequence." Fib. Quart. 15, 323-330, 1977.
Jones, B. W. "A Variation of a Problem of Davenport and Diophantus." Quart. J. Math. (Oxford) Ser. (2) 27, 349-353, 1976.
Kanagasabapathy, P. and Ponnudurai, T. "The Simultaneous Diophantine Equations 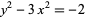 and
and 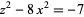 ." Quart. J. Math. (Oxford) Ser. (2) 26, 275-278, 1975.
." Quart. J. Math. (Oxford) Ser. (2) 26, 275-278, 1975.
Morgado, J. "Generalization of a Result of Hoggatt and Bergum on Fibonacci Numbers." Portugaliae Math. 42, 441-445, 1983-1984.
Sansone, G. "Il sistema diofanteo 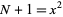 ,
, 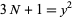 ,
, 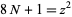 ." Ann. Mat. Pura Appl. 111, 125-151, 1976.
." Ann. Mat. Pura Appl. 111, 125-151, 1976.
Sloane, N. J. A. Sequences A050269, A050269, A050273, A050274, A050275, A051047, and A051048 in "The On-Line Encyclopedia of Integer Sequences."
van Lint, J. H. "On a Set of Diophantine Equations." T. H.-Report 68-WSK-03. Department of Mathematics. Eindhoven, Netherlands: Technological University Eindhoven, 1968.
Referenced on Wolfram|Alpha: Diophantus Property



|
|
|
|
للعاملين في الليل.. حيلة صحية تجنبكم خطر هذا النوع من العمل
|
|
|
|
|
|
|
"ناسا" تحتفي برائد الفضاء السوفياتي يوري غاغارين
|
|
|
|
|
|
|
قسم الشؤون الفكرية ووفد العتبة العلوية المقدسة يبحثان سبل تعزيز التعاون في مجال الفهرسة والتوثيق
|
|
|