
 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 22-11-2018
Date: 25-11-2018
Date: 17-11-2018
|
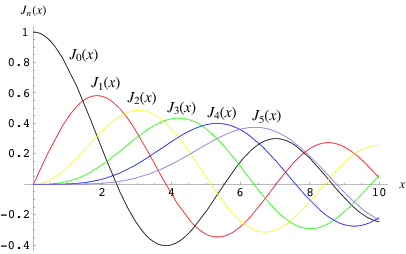
The Bessel functions of the first kind  are defined as the solutions to the Bessel differential equation
are defined as the solutions to the Bessel differential equation
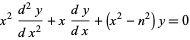 |
(1) |
which are nonsingular at the origin. They are sometimes also called cylinder functions or cylindrical harmonics. The above plot shows  for
for  , 1, 2, ..., 5. The notation
, 1, 2, ..., 5. The notation  was first used by Hansen (1843) and subsequently by Schlömilch (1857) to denote what is now written
was first used by Hansen (1843) and subsequently by Schlömilch (1857) to denote what is now written  (Watson 1966, p. 14). However, Hansen's definition of the function itself in terms of the generating function
(Watson 1966, p. 14). However, Hansen's definition of the function itself in terms of the generating function
 |
(2) |
is the same as the modern one (Watson 1966, p. 14). Bessel used the notation  to denote what is now called the Bessel function of the first kind (Cajori 1993, vol. 2, p. 279).
to denote what is now called the Bessel function of the first kind (Cajori 1993, vol. 2, p. 279).
The Bessel function  can also be defined by the contour integral
can also be defined by the contour integral
 |
(3) |
where the contour encloses the origin and is traversed in a counterclockwise direction (Arfken 1985, p. 416).
The Bessel function of the first kind is implemented in the Wolfram Language as BesselJ[nu, z].
To solve the differential equation, apply Frobenius method using a series solution of the form
 |
(4) |
Plugging into (1) yields
 |
(5) |
 |
(6) |
The indicial equation, obtained by setting  , is
, is
![a_0[k(k-1)+k-m^2]=a_0(k^2-m^2)=0.](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/NumberedEquation7.gif) |
(7) |
Since  is defined as the first nonzero term,
is defined as the first nonzero term,  , so
, so  . Now, if
. Now, if  ,
,
 |
(8) |
 |
(9) |
 |
(10) |
 |
(11) |
First, look at the special case  , then (11) becomes
, then (11) becomes
![sum_(n=2)^infty[a_nn(n-1)+a_(n-2)]x^(m+n)=0,](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/NumberedEquation12.gif) |
(12) |
so
 |
(13) |
Now let  , where
, where  , 2, ....
, 2, ....
 |
 |
 |
(14) |
 |
 |
 |
(15) |
 |
 |
 |
(16) |
which, using the identity  , gives
, gives
 |
(17) |
Similarly, letting  ,
,
 |
(18) |
which, using the identity  , gives
, gives
 |
(19) |
Plugging back into (◇) with  gives
gives
 |
 |
 |
(20) |
 |
 |
![x^(-1/2)[sum_(n=1,3,5,...)^(infty)a_nx^n+sum_(n=0,2,4,...)^(infty)a_nx^n]](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/Inline34.gif) |
(21) |
 |
 |
![x^(-1/2)[sum_(l=0)^(infty)a_(2l)x^(2l)+sum_(l=0)^(infty)a_(2l+1)x^(2l+1)]](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/Inline37.gif) |
(22) |
 |
 |
![x^(-1/2)[a_0sum_(l=0)^(infty)((-1)^l)/((2l)!)x^(2l)+a_1sum_(l=0)^(infty)((-1)^l)/((2l+1)!)x^(2l+1)]](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/Inline40.gif) |
(23) |
 |
 |
 |
(24) |
The Bessel functions of order  are therefore defined as
are therefore defined as
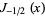 |
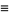 |
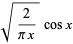 |
(25) |
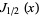 |
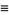 |
 |
(26) |
so the general solution for  is
is
 |
(27) |
Now, consider a general  . Equation (◇) requires
. Equation (◇) requires
 |
(28) |
![[a_nn(2m+n)+a_(n-2)]x^(m+n)=0](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/NumberedEquation19.gif) |
(29) |
for  , 3, ..., so
, 3, ..., so
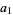 |
 |
 |
(30) |
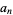 |
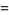 |
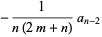 |
(31) |
for  , 3, .... Let
, 3, .... Let  , where
, where  , 2, ..., then
, 2, ..., then
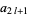 |
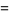 |
![-1/((2l+1)[2(m+l)+1])a_(2l-1)](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/Inline65.gif) |
(32) |
 |
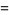 |
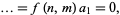 |
(33) |
where  is the function of
is the function of  and
and  obtained by iterating the recursion relationship down to
obtained by iterating the recursion relationship down to  . Now let
. Now let  , where
, where  , 2, ..., so
, 2, ..., so
 |
 |
 |
(34) |
 |
 |
 |
(35) |
 |
 |
 |
(36) |
Plugging back into (◇),
 |
 |
 |
(37) |
 |
 |
 |
(38) |
 |
 |
![a_0sum_(l=0)^(infty)((-1)^l)/([4l(m+l)][4(l-1)(m+l-1)]...[4(m+1)])x^(2l+m)](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/Inline92.gif) |
(39) |
 |
 |
![a_0sum_(l=0)^(infty)([(-1)^lm(m-1)...1]x^(2l+m))/([4l(m+l)][4(l-1)(m+l-1)]...[4(m+1)m...1])](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/Inline95.gif) |
(40) |
 |
 |
 |
(41) |
Now define
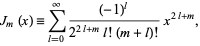 |
(42) |
where the factorials can be generalized to gamma functions for nonintegral  . The above equation then becomes
. The above equation then becomes
 |
(43) |
Returning to equation (◇) and examining the case  ,
,
![a_1(1-2m)+sum_(n=2)^infty[a_nn(n-2m)+a_(n-2)]x^(n-m)=0.](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/NumberedEquation22.gif) |
(44) |
However, the sign of  is arbitrary, so the solutions must be the same for
is arbitrary, so the solutions must be the same for  and
and  . We are therefore free to replace
. We are therefore free to replace  with
with  , so
, so
![a_1(1+2|m|)+sum_(n=2)^infty[a_nn(n+2|m|)+a_(n-2)]x^(|m|+n)=0,](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/NumberedEquation23.gif) |
(45) |
and we obtain the same solutions as before, but with  replaced by
replaced by  .
.
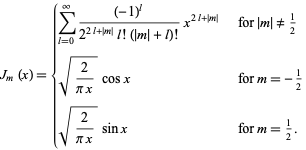 |
(46) |
We can relate  and
and  (when
(when  is an integer) by writing
is an integer) by writing
 |
(47) |
Now let  . Then
. Then
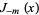 |
 |
 |
(48) |
 |
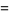 |
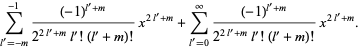 |
(49) |
But  for
for  , so the denominator is infinite and the terms on the left are zero. We therefore have
, so the denominator is infinite and the terms on the left are zero. We therefore have
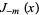 |
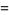 |
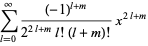 |
(50) |
 |
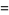 |
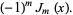 |
(51) |
Note that the Bessel differential equation is second-order, so there must be two linearly independent solutions. We have found both only for  . For a general nonintegral order, the independent solutions are
. For a general nonintegral order, the independent solutions are  and
and  . When
. When  is an integer, the general (real) solution is of the form
is an integer, the general (real) solution is of the form
 |
(52) |
where  is a Bessel function of the first kind,
is a Bessel function of the first kind,  (a.k.a.
(a.k.a.  ) is the Bessel function of the second kind (a.k.a. Neumann function or Weber function), and
) is the Bessel function of the second kind (a.k.a. Neumann function or Weber function), and  and
and  are constants. Complex solutions are given by the Hankel functions (a.k.a. Bessel functions of the third kind).
are constants. Complex solutions are given by the Hankel functions (a.k.a. Bessel functions of the third kind).
The Bessel functions are orthogonal in ![[0,a]](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/Inline135.gif) according to
according to
![int_0^aJ_nu(alpha_(num)rho/a)J_nu(alpha_(nun)rho/a)rhodrho=1/2a^2[J_(nu+1)(alpha_(num))]^2delta_(mn),](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/NumberedEquation27.gif) |
(53) |
where  is the
is the  th zero of
th zero of  and
and  is the Kronecker delta (Arfken 1985, p. 592).
is the Kronecker delta (Arfken 1985, p. 592).
Except when  is a negative integer,
is a negative integer,
 |
(54) |
where  is the gamma function and
is the gamma function and  is a Whittaker function.
is a Whittaker function.
In terms of a confluent hypergeometric function of the first kind, the Bessel function is written
 |
(55) |
A derivative identity for expressing higher order Bessel functions in terms of  is
is
 |
(56) |
where  is a Chebyshev polynomial of the first kind. Asymptotic forms for the Bessel functions are
is a Chebyshev polynomial of the first kind. Asymptotic forms for the Bessel functions are
 |
(57) |
for  and
and
 |
(58) |
for  (correcting the condition of Abramowitz and Stegun 1972, p. 364).
(correcting the condition of Abramowitz and Stegun 1972, p. 364).
A derivative identity is
![d/(dx)[x^mJ_m(x)]=x^mJ_(m-1)(x).](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/NumberedEquation33.gif) |
(59) |
An integral identity is
 |
(60) |
Some sum identities are
 |
(61) |
(which follows from the generating function (◇) with  ),
),
![1=[J_0(x)]^2+2sum_(k=1)^infty[J_k(x)]^2](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/NumberedEquation36.gif) |
(62) |
(Abramowitz and Stegun 1972, p. 363),
 |
(63) |
(Abramowitz and Stegun 1972, p. 361),
 |
(64) |
for  (Abramowitz and Stegun 1972, p. 361),
(Abramowitz and Stegun 1972, p. 361),
 |
(65) |
(Abramowitz and Stegun 1972, p. 361), and the Jacobi-Anger expansion
 |
(66) |
which can also be written
 |
(67) |
The Bessel function addition theorem states
 |
(68) |
Various integrals can be expressed in terms of Bessel functions
 |
(69) |
which is Bessel's first integral,
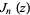 |
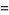 |
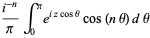 |
(70) |
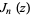 |
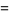 |
 |
(71) |
for  , 2, ...,
, 2, ...,
 |
(72) |
for  , 2, ...,
, 2, ...,
 |
(73) |
for  . The Bessel functions are normalized so that
. The Bessel functions are normalized so that
 |
(74) |
for positive integral (and real)  . Integrals involving
. Integrals involving  include
include
![int_0^infty[(J_1(x))/x]^2dx=4/(3pi)](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/NumberedEquation47.gif) |
(75) |
![int_0^infty[(J_1(x))/x]^2xdx=1/2.](http://mathworld.wolfram.com/images/equations/BesselFunctionoftheFirstKind/NumberedEquation48.gif) |
(76) |
Ratios of Bessel functions of the first kind have continued fraction
 |
(77) |
(Wall 1948, p. 349).
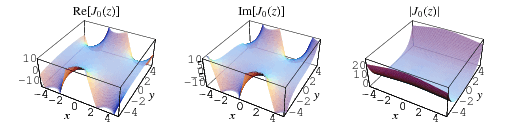
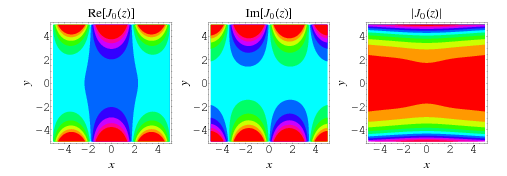
The special case of  gives
gives  as the series
as the series
 |
(78) |
(Abramowitz and Stegun 1972, p. 360), or the integral
 |
(79) |
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). "Bessel Functions  and
and 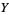 ." §9.1 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 358-364, 1972.
." §9.1 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 358-364, 1972.
Arfken, G. "Bessel Functions of the First Kind, 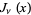 " and "Orthogonality." §11.1 and 11.2 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 573-591 and 591-596, 1985.
" and "Orthogonality." §11.1 and 11.2 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 573-591 and 591-596, 1985.
Cajori, F. A History of Mathematical Notations, Vols. 1-2. New York: Dover, 1993.
Hansen, P. A. "Ermittelung der absoluten Störungen in Ellipsen von beliebiger Excentricität und Neigung, I." Schriften der Sternwarte Seeberg. Gotha, 1843.
Lehmer, D. H. "Arithmetical Periodicities of Bessel Functions." Ann. Math. 33, 143-150, 1932.
Le Lionnais, F. Les nombres remarquables. Paris: Hermann, 1983.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 619-622, 1953.
Schlömilch, O. X. "Ueber die Bessel'schen Function." Z. für Math. u. Phys. 2, 137-165, 1857.
Spanier, J. and Oldham, K. B. "The Bessel Coefficients  and
and 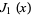 " and "The Bessel Function
" and "The Bessel Function  ." Chs. 52-53 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 509-520 and 521-532, 1987.
." Chs. 52-53 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 509-520 and 521-532, 1987.
Wall, H. S. Analytic Theory of Continued Fractions. New York: Chelsea, 1948.
Watson, G. N. A Treatise on the Theory of Bessel Functions, 2nd ed. Cambridge, England: Cambridge University Press, 1966.



|
|
|
|
5 علامات تحذيرية قد تدل على "مشكل خطير" في الكبد
|
|
|
|
|
|
|
لحماية التراث الوطني.. العتبة العباسية تعلن عن ترميم أكثر من 200 وثيقة خلال عام 2024
|
|
|