Gauss-Kuzmin Distribution
المؤلف:
Babenko, K. I.
المصدر:
"On a Problem of Gauss." Soviet Math. Dokl. 19
الجزء والصفحة:
...
2-5-2020
1045
Gauss-Kuzmin Distribution
The Gauss-Kuzmin distribution is the distribution of occurrences of a positive integer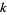 
in the continued fraction of a random (or "generic") real number.
Consider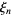 
defined for a real number 
by
so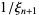 
is the fractional part of 
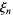. This can be defined recursively through
 |
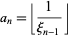(3)
|
and
 |
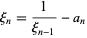(4)
|
with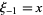 
and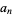 
simply the 
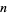th term of the continued fraction ![x=[a_0;a_1,...]](https://mathworld.wolfram.com/images/equations/Gauss-KuzminDistribution/Inline15.gif)
.
The distribution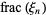 
was considered by Gauss in a letter to Laplace dated January 30, 1812. In this letter, Gauss said that he could prove by a simple argument that if 
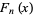, sometimes denoted 
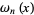(Havil 2003, p. 156), is the probability that 
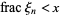for a random 
, then
 |
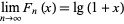(5)
|
(Rockett and Szüsz 1992, pp. 151-152; Knuth 1998, p. 341; Havil 2003, p. 157). Histograms of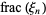 
are illustrated above for 5000 terms of 
, the Euler-Mascheroni constant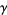 
, Catalan's constant 
, and the natural logarithm of 2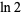 
.
However, Gauss was unable to describe the behavior of the correction term in
 |
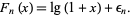(6)
|
Kuz'min (1928) published the first analysis of the asymptotic behavior of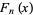 
, obtaining
 |
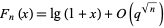(7)
|
with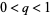 
. Using a different method, Lévy (1929) obtained
 |
(8)
|
with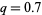 
. Wirsing (1974) subsequently showed, among other results, that
 |
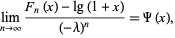(9)
|
where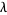 
is a constant known as Gauss-Kuzmin-Wirsing constant and 
is an analytic function with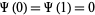 
.
It follows from Gauss's result that
(Bailey et al. 1997; Havil 2003, p. 158), where 
and "Kuzmin" is sometimes also written as "Kuz'min." The plot above shows the distribution of the first 500 terms in the continued fractions of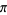 
, 
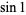, the Euler-Mascheroni constant 
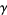, and the Copeland-Erdős constant 
. The distribution is properly normalized, since
![-sum_(k=1)^inftylg[1-1/((k+1)^2)]=1.](https://mathworld.wolfram.com/images/equations/Gauss-KuzminDistribution/NumberedEquation8.gif) |
(12)
|
REFERENCES:
Babenko, K. I. "On a Problem of Gauss." Soviet Math. Dokl. 19, 136-140, 1978.
Bailey, D. H.; Borwein, J. M.; and Crandall, R. E. "On the Khintchine Constant." Math. Comput. 66, 417-431, 1997.
Daudé, H.; Flajolet, P.; and Vallé, B. "An Average-Case Analysis of the Gaussian Algorithm for Lattice Reduction." Combin. Probab. Comput. 6, 397-433, 1997.
Durner, A. "On a Theorem of Gauss-Kuzmin-Lévy." Arch. Math. 58, 251-256, 1992.
Finch, S. R. "Gauss-Kuzmin-Wirsing Constant." §2.17 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 151-156, 2003.
Havil, J. Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, pp. 155-159, 2003.
Khinchin, A. Ya. "Gauss's Problem and Kuz'min's Theorem." §15 in Continued Fractions. New York: Dover, pp. 71-86, 1997.
Knuth, D. E. The Art of Computer Programming, Vol. 2: Seminumerical Algorithms, 3rd ed. Reading, MA: Addison-Wesley, 1998.
Kuz'min, R. O. "A Problem of Gauss." Dokl. Akad. Nauk, Ser. A, 375-380, 1928.
Kuz'min, R. O. "Sur un problème de Gauss." Anni Congr. Intern. Bologne 6, 83-89, 1928.
Lévy, P. "Sur les lois de probabilité dont dependent les quotients complets et incomplets d'une fraction continue." Bull. Soc. Math. France 57, 178-194, 1929.
Lévy, P. "Sur les lois de probabilité dont dependent les quotients complets et incomplets d'une fraction continue." Bull. Soc. Math. France 57, 178-194, 1929.
Rockett, A. M. and Szüsz, P. "The Gauss-Kuzmin Theorem." §5.5 in Continued Fractions. New York: World Scientific, pp. 151-155, 1992.
Wirsing, E. "On the Theorem of Gauss-Kuzmin-Lévy and a Frobenius-Type Theorem for Function Spaces." Acta Arith. 24, 507-528, 1974.
الاكثر قراءة في نظرية الاعداد
اخر الاخبار
اخبار العتبة العباسية المقدسة
