An obtuse triangle is a triangle in which one of the angles is an obtuse angle. (Obviously, only a single angle in a triangle can be obtuse or it wouldn't be a triangle.) A triangle must be either obtuse, acute, or right.

From the law of cosines, for a triangle with side lengths 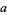, , 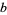and ,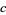

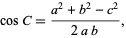(1)

with 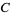 the angle opposite side 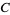. For an angle to be obtuse, 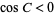. Therefore, an obtuse triangle satisfies one of 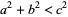, , 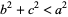or .

An obtuse triangle can be dissected into no fewer than seven acute triangles (Wells 1986, p. 71).

A famous problem is to find the chance that three points picked randomly in a plane are the polygon vertices of an obtuse triangle (Eisenberg and Sullivan 1996). Unfortunately, the solution of the problem depends on the procedure used to pick the "random" points (Portnoy 1994). In fact, it is impossible to pick random variables which are uniformly distributed in the plane (Eisenberg and Sullivan 1996). Guy (1993) gives a variety of solutions to the problem. Woolhouse (1886) solved the problem by picking uniformly distributed points in the unit disk, and obtained

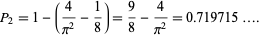(2)

The problem was generalized by Hall (1982) to -dimensional ball triangle picking, and Buchta (1986) gave closed form evaluations for Hall's integrals.

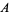In 1893, Lewis Carroll (1976) posed and gave another solution to the problem as follows. Call the longest side of a triangle , and call the diameter . Draw arcs from  and 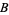 of radius 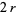. Because the longest side of the triangle is defined to be 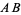, the third polygon vertex of the triangle must lie within the region 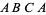. If the third polygon vertex lies within the semicircle, the triangle is an obtuse triangle. If the polygon vertex lies on the semicircle (which will happen with probability 0), the triangle is a right triangle. Otherwise, it is an acute triangle. The chance of obtaining an obtuse triangle is then the ratio of the area of the semicircle to that of 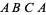. The area of  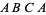is then twice the area of a circular sector minus the area of the triangle.

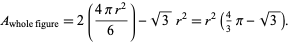(3)

Therefore,

(4)

REFERENCES:

Buchta, C. "A Note on the Volume of a Random Polytope in a Tetrahedron." Ill. J. Math. 30, 653-659, 1986.

Carroll, L. Pillow Problems & A Tangled Tale. New York: Dover, 1976.

Eisenberg, B. and Sullivan, R. "Random Triangles 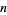 Dimensions." Amer. Math. Monthly 103, 308-318, 1996.

Guy, R. K. "There are Three Times as Many Obtuse-Angled Triangles as There are Acute-Angled Ones." Math. Mag. 66, 175-178, 1993.

Hall, G. R. "Acute Triangles in the -Ball." J. Appl. Prob. 19, 712-715, 1982.

Portnoy, S. "A Lewis Carroll Pillow Problem: Probability on at Obtuse Triangle." Statist. Sci. 9, 279-284, 1994.

Wells, D. The Penguin Dictionary of Curious and Interesting Numbers. Middlesex, England: Penguin Books, p. 71, 1986.

Wells, D. G. The Penguin Book of Interesting Puzzles. London: Penguin Books, pp. 67 and 248-249, 1992.

Woolhouse, W. S. B. Solution to Problem 1350. Mathematical Questions, with Their Solutions, from the Educational Times, 1. London: F. Hodgson and Son, 49-51, 1886.

اخر الاخبار

اخبار العتبة العباسية المقدسة

المجمَع العلمي يقيم مسابقة الكوثر الخاصة بترتيل القرآن الكريم في كربلاء

قسم الشؤون الفكرية والثقافية يُصدر العدد الثالث والعشرين من مجلّة (أوراق معرفية)

قسم التربية والتعليم يُطلق الموسم التدريبيّ الصيفيّ لملاكات مجموعة العميد التربوية

المجمَع العلمي يُطلق المرحلة الثالثة من مشروع أمير القرّاء الوطني

المزيد

الآخبار الصحية

اختراق علمي.. دواء يؤخر ظهور السكري من النوع الأول لسنوات

هذا ما يحدث لجسمك إن أهملت نظافة فراشك

لماذا تعتبر النساء أكثر عرضة للإصابة بألزهايمر من الرجال؟

لماذا يحذر الخبراء من الإفراط في تناول الفراولة؟

المزيد

الآخبار التكنلوجية

السيارات الكهربائية أنظف بنسبة 73% من سيارات الوقود

سيارة لوسِد الكهربائية تدخل "غينيس" بأطول مدى في العالم

نظرية جديدة تنسف كل ما نعرفه عن تشكل أول قارة في الأرض

منذ 37 ألف عام.. دراسة تكشف "أقدم أمراض" أصابت البشر

المزيد