Wilf-Zeilberger Pair
المؤلف:
Amdeberhan, T. and Zeilberger, D.
المصدر:
"Hypergeometric Series Acceleration via the WZ Method." Electronic J.
الجزء والصفحة:
...
22-6-2019
2780
Wilf-Zeilberger Pair
A pair of closed form functions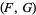 
is said to be a Wilf-Zeilberger pair if
 |
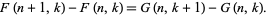(1)
|
The Wilf-Zeilberger formalism provides succinct proofs of known identities and allows new identities to be discovered whenever it succeeds in finding a proof certificate for a known identity. However, if the starting point is an unknown hypergeometric sum, then the Wilf-Zeilberger method cannot discover a closed form solution, while Zeilberger's algorithm can.
Wilf-Zeilberger pairs are very useful in proving hypergeometric identities of the form
 |
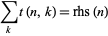(2)
|
for which the addend 
vanishes for all 
outside some finite interval. Now divide by the right-hand side to obtain
 |
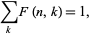(3)
|
where
 |
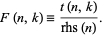(4)
|
Now use a rational function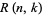 
provided by Zeilberger's algorithm, define
 |
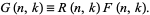(5)
|
The identity (◇) then results. Summing the relation over all integers then telescopes the right side to 0, giving
 |
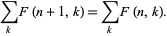(6)
|
Therefore,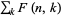 
is independent of 
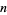, and so must be a constant. If 
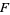is properly normalized, then it will be true that 
.
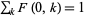For example, consider the binomial coefficient identity
 |
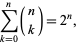(7)
|
the function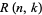 
returned by Zeilberger's algorithm is
 |
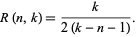(8)
|
Therefore,
 |
(9)
|
and
Taking
 |
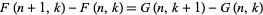(14)
|
then gives the alleged identity
 |
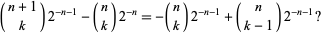(15)
|
Expanding and evaluating shows that the identity does actually hold, and it can also be verified that
![F(0,k)=(0; k)=<span style=]() {1 for k=0; 0 otherwise, " src="http://mathworld.wolfram.com/images/equations/Wilf-ZeilbergerPair/NumberedEquation12.gif" style="height:41px; width:192px" /> |
(16)
|
so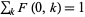 
(Petkovšek et al. 1996, pp. 25-27).
For any Wilf-Zeilberger pair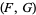 
,
![sum_(n=0)^inftyG(n,0)=sum_(n=1)^infty[F(n,n-1)+G(n-1,n-1)]](http://mathworld.wolfram.com/images/equations/Wilf-ZeilbergerPair/NumberedEquation13.gif) |
(17)
|
whenever either side converges (Zeilberger 1993). In addition,
![sum_(n=0)^inftyG(n,0)=sum_(n=0)^infty[F(s(n+1),n)+sum_(i=0)^(s-1)G(sn+i,n)]
-lim_(n->infty)sum_(k=0)^(n-1)F(sn,k)](http://mathworld.wolfram.com/images/equations/Wilf-ZeilbergerPair/NumberedEquation14.gif) |
(18)
|
 |
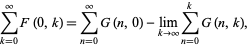(19)
|
and
![sum_(n=0)^inftyG(n,0)=sum_(n=0)^infty[sum_(j=0)^(t-1)F(s(n+1),tn+j)+sum_(i=0)^(s-1)G(sn+i,tn)]-lim_(n->infty)sum_(k=0)^(n-1)F_(s,t)(n,k),](http://mathworld.wolfram.com/images/equations/Wilf-ZeilbergerPair/NumberedEquation16.gif) |
(20)
|
where
(Amdeberhan and Zeilberger 1997). The latter identity has been used to compute Apéry's constant to a large number of decimal places (Wedeniwski).
REFERENCES:
Amdeberhan, T. and Zeilberger, D. "Hypergeometric Series Acceleration via the WZ Method." Electronic J. Combinatorics 4, No. 2, R3, 1-3, 1997. http://www.combinatorics.org/Volume_4/Abstracts/v4i2r3.html. Also available at http://www.math.temple.edu/~zeilberg/mamarim/mamarimhtml/accel.html.
Bailey, D. H.; Borwein, J. M.; Calkin, N. J.; Girgensohn, R.; Luke, D. R.; and Moll, V. H. "The Wilf-Zeilberger Algorithm." §3.1 in Experimental Mathematics in Action. Wellesley, MA: A K Peters, pp. 53-55, 2007.
Cipra, B. A. "How the Grinch Stole Mathematics." Science 245, 595, 1989.
Koepf, W. "Algorithms for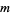 
-fold Hypergeometric Summation." J. Symb. Comput. 20, 399-417, 1995.
Koepf, W. "The Wilf-Zeilberger Method." Ch. 6 in Hypergeometric Summation: An Algorithmic Approach to Summation and Special Function Identities. Braunschweig, Germany: Vieweg, pp. 80-92, 1998.
Petkovšek, M.; Wilf, H. S.; and Zeilberger, D. "The WZ Phenomenon." Ch. 7 in A=B. Wellesley, MA: A K Peters, pp. 121-140, 1996. http://www.cis.upenn.edu/~wilf/AeqB.html.
Wedeniwski, S. "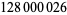
Digits of Zeta(3)." http://pi.lacim.uqam.ca/eng/records_en.html.
Wilf, H. S. and Zeilberger, D. "Rational Functions Certify Combinatorial Identities." J. Amer. Math. Soc. 3, 147-158, 1990.
Zeilberger, D. "The Method of Creative Telescoping." J. Symb. Comput. 11, 195-204, 1991.
Zeilberger, D. "Closed Form (Pun Intended!)." Contemporary Math. 143, 579-607, 1993.
الاكثر قراءة في التفاضل و التكامل
اخر الاخبار
اخبار العتبة العباسية المقدسة
