Confluent Hypergeometric Function of the Second Kind
المؤلف:
Abramowitz, M. and Stegun, I. A.
المصدر:
"Confluent Hypergeometric Functions." Ch. 13 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover
الجزء والصفحة:
...
10-6-2019
4578
Confluent Hypergeometric Function of the Second Kind
The confluent hypergeometric function of the second kind gives the second linearly independent solution to the confluent hypergeometric differential equation. It is also known as the Kummer's function of the second kind, Tricomi function, or Gordon function. It is denoted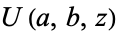 
and can be defined by
where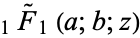 
is a regularized confluent hypergeometric function of the first kind, 
is a gamma function, and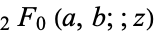 
is a generalized hypergeometric function (which converges nowhere but exists as a formal power series; Abramowitz and Stegun 1972, p. 504).
It has an integral representation
 |
(3)
|
for ![R[a],R[z]>0](http://mathworld.wolfram.com/images/equations/ConfluentHypergeometricFunctionoftheSecondKind/Inline11.gif)
(Abramowitz and Stegun 1972, p. 505).
The confluent hypergeometric function of the second kind is implemented in the Wolfram Language as HypergeometricU[a, b, z].
The Whittaker functions give an alternative form of the solution.
The function has a Maclaurin series
 |
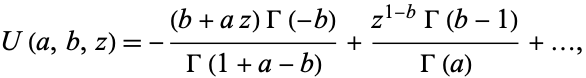(4)
|
and asymptotic series
![U(a,b,z)∼(1/z)^a[1+a(b-a-1)z^(-1)
+1/2a(a+1)(a+b-1)(2+b-a)z^(-2)+...].](http://mathworld.wolfram.com/images/equations/ConfluentHypergeometricFunctionoftheSecondKind/NumberedEquation3.gif) |
(5)
|
has derivative
 |
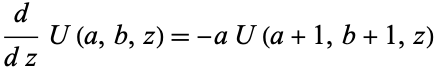(6)
|
and indefinite integral
 |
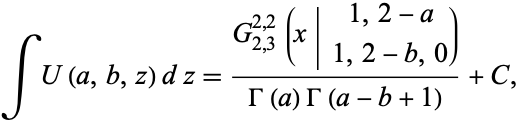(7)
|
where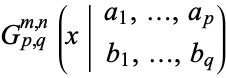 
is a Meijer G-function and 
is a constant of integration.
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). "Confluent Hypergeometric Functions." Ch. 13 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 503-515, 1972.
Arfken, G. "Confluent Hypergeometric Functions." §13.6 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 753-758, 1985.
Buchholz, H. The Confluent Hypergeometric Function with Special Emphasis on its Applications. New York: Springer-Verlag, 1969.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 671-672, 1953.
Slater, L. J. "The Second Form of Solutions of Kummer's Equations." §1.3 in Confluent Hypergeometric Functions. Cambridge, England: Cambridge University Press, p. 5, 1960.
Spanier, J. and Oldham, K. B. "The Tricomi Function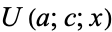 
." Ch. 48 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 471-477, 1987.
الاكثر قراءة في التفاضل و التكامل
اخر الاخبار
اخبار العتبة العباسية المقدسة
