Complex Number

The complex numbers are the field 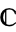 of numbers of the form 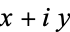, where  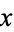and 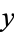 are real numbers and i is the imaginary unit equal to the square root of 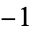, 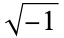. When a single letter  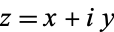is used to denote a complex number, it is sometimes called an "affix." In component notation,  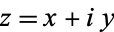can be written 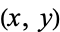. The field of complex numbers includes the field of real numbers as a subfield.

The set of complex numbers is implemented in the Wolfram Language as Complexes. A number 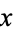 can then be tested to see if it is complex using the command Element[x, Complexes], and expressions that are complex numbers have the Head of Complex.

Complex numbers are useful abstract quantities that can be used in calculations and result in physically meaningful solutions. However, recognition of this fact is one that took a long time for mathematicians to accept. For example, John Wallis wrote, "These Imaginary Quantities (as they are commonly called) arising from the Supposed Root of a Negative Square (when they happen) are reputed to imply that the Case proposed is Impossible" (Wells 1986, p. 22).

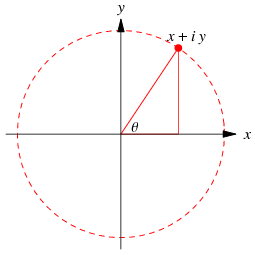
Through the Euler formula, a complex number

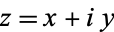(1)

may be written in "phasor" form

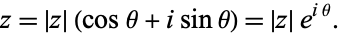(2)

Here, 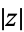 is known as the complex modulus (or sometimes the complex norm) and  is known as the complex argument or phase. The plot above shows what is known as an Argand diagram of the point 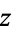, where the dashed circle represents the complex modulus  of 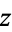 and the angle  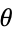represents its complex argument. Historically, the geometric representation of a complex number as simply a point in the plane was important because it made the whole idea of a complex number more acceptable. In particular, "imaginary" numbers became accepted partly through their visualization.

Unlike real numbers, complex numbers do not have a natural ordering, so there is no analog of complex-valued inequalities. This property is not so surprising however when they are viewed as being elements in the complex plane, since points in a plane also lack a natural ordering.

The absolute square of 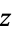 is defined by 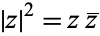, with  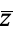the complex conjugate, and the argument may be computed from

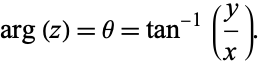(3)

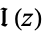The real  and imaginary parts  are given by

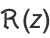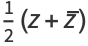			(4)
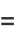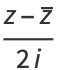			(5)
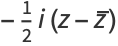			(6)
			(7)

de Moivre's identity relates powers of complex numbers for real 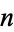 by

(8)

A power of complex number 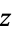 to a positive integer exponent  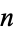can be written in closed form as

(9)

The first few are explicitly

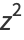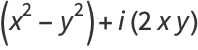			(10)
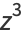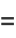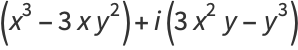			(11)
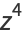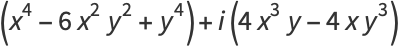			(12)
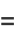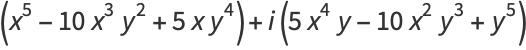			(13)

(Abramowitz and Stegun 1972).

Complex addition

(14)

complex subtraction

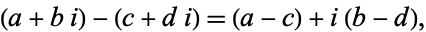(15)

complex multiplication

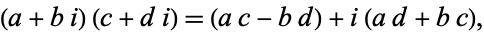(16)

and complex division

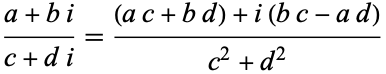(17)

can also be defined for complex numbers. Complex numbers may also be taken to complex powers. For example, complex exponentiation obeys

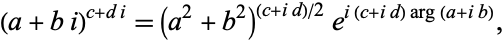(18)

where 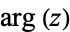 is the complex argument.

REFERENCES:

Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 16-17, 1972.

Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 353-357, 1985.

Bold, B. "Complex Numbers." Ch. 3 in Famous Problems of Geometry and How to Solve Them. New York: Dover, pp. 19-27, 1982.

Courant, R. and Robbins, H. "Complex Numbers." §2.5 in What Is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, pp. 88-103, 1996.

Ebbinghaus, H. D.; Hirzebruch, F.; Hermes, H.; Prestel, A; Koecher, M.; Mainzer, M.; and Remmert, R. Numbers. New York: Springer-Verlag, 1990.

Krantz, S. G. "Complex Arithmetic." §1.1 in Handbook of Complex Variables. Boston, MA: Birkhäuser, pp. 1-7, 1999.

Mazur, B. Imagining Numbers (Particularly the Square Root of Minus Fifteen). Farrar, Straus and Giroux, 2003.

Morse, P. M. and Feshbach, H. "Complex Numbers and Variables." §4.1 in Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 349-356, 1953.

Nahin, P. J. An Imaginary Tale: The Story of -1. Princeton, NJ: Princeton University Press, 2007.

Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. "Complex Arithmetic." §5.4 in Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England: Cambridge University Press, pp. 171-172, 1992.

Wells, D. The Penguin Dictionary of Curious and Interesting Numbers. Middlesex, England: Penguin Books, pp. 21-23, 1986.

Wolfram, S. A New Kind of Science. Champaign, IL: Wolfram Media, p. 1168, 2002.