The Cauchy principal value of a finite integral of a function 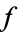 about a point  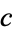with 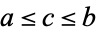 is given by

(Henrici 1988, p. 261; Whittaker and Watson 1990, p. 117; Bronshtein and Semendyayev 1997, p. 283). Similarly, the Cauchy principal value of a doubly infinite integral of a function  is defined by

The Cauchy principal value is also known as the principal value integral (Henrici 1988, p. 261), finite part (Vladimirov 1971), or partie finie (Vladimirov 1971).

The Cauchy principal value of an integral having no nonsimple poles can be computed in the Wolfram Language using Integrate[f, {" src="http://mathworld.wolfram.com/images/equations/CauchyPrincipalValue/Inline5.gif" style="height:14px; width:5px" />x, a, b}" src="http://mathworld.wolfram.com/images/equations/CauchyPrincipalValue/Inline6.gif" style="height:14px; width:5px" />, PrincipalValue -> True]. Cauchy principal values of functions with possibly nonsimple poles can be computed numerically using the "CauchyPrincipalValue" method in NIntegrate.

Cauchy principal values are important in the theory of generalized functions, where they allow extension of 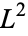 results to .

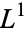Cauchy principal values are sometimes simply known as "principal values" (e.g., Vladimirov 1971, p. 75) even though they are not related to the principal value of complex analysis.

The most common designation for the Cauchy principal values seems to be 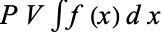 (Henrici 1988, pp. 259-262; Gradshteyn and Ryzhik 2000, p. 523). Sometimes, no explicit designation is used (Harris and Stocker 1998, p. 552; Gradshteyn and Ryzhik 2000, p. 248). Other notations include  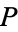(Arfken 1985, p. 403), P.V. (Apelblat 1983, p. viii),  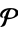(Morse and Feshbach 1953, p. 368; most Russian authors),  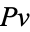(Vladimirov 1971),  (Bronshtein and Semendyayev 1997, p. 282), and V.P. (Brychkov 1992, p. 7). For integrals with finite limits, the Cauchy principal value is sometimes denoted  (Zwillinger 1995, p. 346).

REFERENCES:

Apelblat, A. Table of Definite and Indefinite Integrals. Amsterdam, Netherlands: Elsevier, 1983.

Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 401-403, 1985.

Bronshtein, I. N. and Semendyayev, K. A. Handbook of Mathematics, 3rd ed. New York: Springer-Verlag, 1997.

Brychkov, Yu. A.; Glaeske, H.-J.; Prudnikov, A. P.; and Tuan, V. K. Multidimensional Integral Transformations. Philadelphia, PA: Gordon and Breach, 1992.

Cauchy, A. "Sur un nouveau genre de calcul analogue au calcul infinitésimal." Exercises de mathematiques 1826. Reprinted in Oeuvres complètes, Ser. 2, Vol. 6. Paris: Gauthier-Villars, pp. 23-37, 1882-1974.

Dieudonné, J. Geschichte der Mathematik 1700-1900: Ein Abriß. Berlin: VEB Deutscher Verlag der Wissenschaften, p. 149, 1985.

Gradshteyn, I. S. and Ryzhik, I. M. "The Principal Values of Improper Integrals." §3.05 in Tables of Integrals, Series, and Products, 6th ed. San Diego, CA: Academic Press, p. 248, 2000.

Harris, J. W. and Stocker, H. Handbook of Mathematics and Computational Science. New York: Springer-Verlag, 1998.

Henrici, P. Applied and Computational Complex Analysis, Vol. 1: Power Series, Integration, Conformal Mapping, Location of Zeros. New York: Wiley, 1988.

Maurin, K. Analysis: Part Two: Integration, Distributions, Holomorphic Functions, Tensor and Harmonic Analysis. Amsterdam, Netherlands: Kluwer, 2001.

Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, 1953.

Sansone, G. Orthogonal Functions, rev. English ed. New York: Dover, p. 158, 1991.

Vladimirov, V. S. Equations of Mathematical Physics. New York: Dekker, 1971.

Whittaker, E. T. and Watson, G. N. A Course in Modern Analysis, 4th ed. Cambridge, England: Cambridge University Press, 1990.

Zwillinger, D. (Ed.). CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, 1995.

اخر الاخبار

اخبار العتبة العباسية المقدسة

العتبة العباسية المقدسة تكرم المشاركين في مشروع مقرأة العميد الرقمية

تقديم محاضرة عن عالم الأرقام في القرآن الكريم ضمن حفل إطلاق مشروع مقرأة العميد

ضمن فعاليات إطلاق مقرأة العميد الرقمية.. عرض فيديو توثيقي عن فكرة المشروع

جامعة تكنولوجيا المعلومات: مشروع مقرأة العميد منصة رقمية متكاملة لتعليم القرآن الكريم وتلاوته وحفظه

المزيد

الآخبار الصحية

عرض شائع على الأصابع.. قد يكشف سرطان الرئة مبكرا

7 مشروبات طبيعية لتقليل التوتر والقلق

دراسة: "خطر مبكر" يهدد صحة الأطفال

الجوز أو الفول السوداني.. أيهما يتفوّق في حماية القلب؟

المزيد

الآخبار التكنلوجية

مفاجأة علمية عن الإنجاب.. كم طفلا يطيل عمر المرأة؟

استطلاع يكشف "الأكثر قلقا" من تأثر الوظائف بالذكاء الاصطناعي

هل يمنحك تناول الجزر يوميا سمرة طبيعية؟

إنجاز طبي تاريخي.. جراحة قلب بلا شق في الصدر

المزيد