

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Distinct Prime Factors
المؤلف:
Abramowitz, M. and Stegun, I. A.
المصدر:
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover
الجزء والصفحة:
...
12-9-2020
2282
Distinct Prime Factors
The distinct prime factors of a positive integer  are defined as the
are defined as the 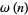 numbers
numbers 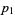 , ...,
, ...,  in the prime factorization
in the prime factorization
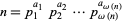 |
(1) |
(Hardy and Wright 1979, p. 354).
A list of distinct prime factors of a number  can be computed in the Wolfram Language using FactorInteger[n][[All, 1]], and the number
can be computed in the Wolfram Language using FactorInteger[n][[All, 1]], and the number 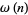 of distinct prime factors is implemented as PrimeNu[n].
of distinct prime factors is implemented as PrimeNu[n].
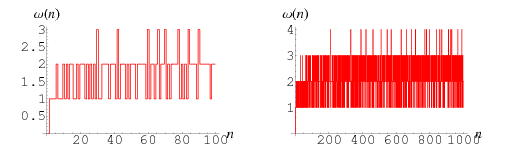
The first few values of  for
for 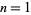 , 2, ... are 0, 1, 1, 1, 1, 2, 1, 1, 1, 2, 1, 2, 1, 2, 2, 1, 1, 2, 1, 2, ... (OEIS A001221; Abramowitz and Stegun 1972, Kac 1959). This sequence is given by the inverse Möbius transform of
, 2, ... are 0, 1, 1, 1, 1, 2, 1, 1, 1, 2, 1, 2, 1, 2, 2, 1, 1, 2, 1, 2, ... (OEIS A001221; Abramowitz and Stegun 1972, Kac 1959). This sequence is given by the inverse Möbius transform of {chi_P(n)}" src="https://mathworld.wolfram.com/images/equations/DistinctPrimeFactors/Inline9.gif" style="height:15px; width:44px" />, where
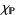 is the characteristic function of the prime numbers (Sloane and Plouffe 1995, p. 22). The prime factorizations and distinct prime factors of the first few positive integers are listed in the table below.
is the characteristic function of the prime numbers (Sloane and Plouffe 1995, p. 22). The prime factorizations and distinct prime factors of the first few positive integers are listed in the table below.
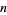 |
prime factorization | 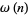 |
distinct prime factors (A027748) |
| 1 | -- | 0 | -- |
| 2 | 2 | 1 | 2 |
| 3 | 3 | 1 | 3 |
| 4 |  |
1 | 2 |
| 5 | 5 | 1 | 5 |
| 6 | 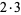 |
2 | 2, 3 |
| 7 | 7 | 1 | 7 |
| 8 | 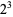 |
1 | 2 |
| 9 | 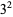 |
1 | 3 |
| 10 |  |
2 | 2, 5 |
| 11 | 11 | 1 | 11 |
| 12 | 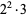 |
2 | 2, 3 |
| 13 | 13 | 1 | 13 |
| 14 | 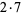 |
2 | 2, 7 |
| 15 | 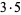 |
2 | 3, 5 |
| 16 | 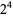 |
1 | 2 |
The numbers consisting only of distinct prime factors are precisely the squarefree numbers.
A sum involving 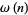 is given by
is given by
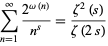 |
(2) |
for 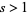 (Hardy and Wright 1979, p. 255).
(Hardy and Wright 1979, p. 255).
The average order of 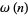 is
is
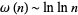 |
(3) |
(Hardy 1999, p. 51). More precisely,
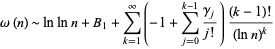 |
(4) |
(Diaconis 1976, Knuth 2000, Diaconis 2002, Finch 2003, Knuth 2003), where 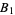 is the Mertens constant and
is the Mertens constant and  are Stieltjes constants. Furthermore, the variance is given by
are Stieltjes constants. Furthermore, the variance is given by
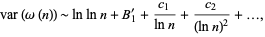 |
(5) |
where
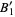 |
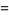 |
 |
(6) |
 |
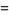 |
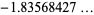 |
(7) |
(OEIS A091588), where
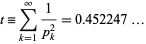 |
(8) |
(OEIS A085548) is the prime zeta function 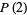 (Finch 2003). The coefficients
(Finch 2003). The coefficients 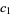 and
and 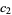 are given by the sums
are given by the sums
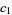 |
 |
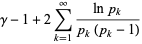 |
(9) |
 |
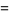 |
 |
(10) |
 |
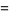 |
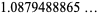 |
(11) |
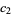 |
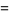 |
![-gamma_1-(gamma-1)[gamma+2sum_(k=1)^(infty)(lnp_k)/(p_k(p_k-1))]+2sum_(k=1)^(infty)((2p_k-1)(lnp_k)^2)/(2p_k(p_k-1)^2)](https://mathworld.wolfram.com/images/equations/DistinctPrimeFactors/Inline47.gif) |
(12) |
 |
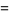 |
 |
(13) |
(Diaconis 1976, Knuth 2000, Diaconis 2002, Finch 2003, Knuth 2003), where
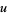 |
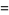 |
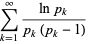 |
(14) |
 |
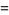 |
 |
(15) |
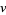 |
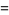 |
 |
(16) |
 |
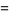 |
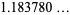 |
(17) |
(Finch 2003).
If 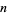 is a primorial, then
is a primorial, then
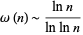 |
(18) |
(Hardy and Wright 1979, p. 355).
The summatory function of 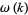 is given by
is given by
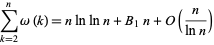 |
(19) |
where 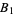 is the Mertens constant (Hardy 1999, p. 57), the
is the Mertens constant (Hardy 1999, p. 57), the 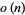 term (Hardy and Ramanujan 1917; Hardy and Wright 1979, p. 355) has been rewritten in a more explicit form, and
term (Hardy and Ramanujan 1917; Hardy and Wright 1979, p. 355) has been rewritten in a more explicit form, and 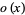 and
and 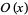 are asymptotic notation. The first few values of the summatory function are 1, 2, 3, 4, 6, 7, 8, 9, 11, 12, 14, 15, 17, 19, 20, 21, ... (OEIS A013939). In addition,
are asymptotic notation. The first few values of the summatory function are 1, 2, 3, 4, 6, 7, 8, 9, 11, 12, 14, 15, 17, 19, 20, 21, ... (OEIS A013939). In addition,
![sum_(k=2)^n[omega(k)]^2=n(lnlnn)^2+O(nlnlnn)](https://mathworld.wolfram.com/images/equations/DistinctPrimeFactors/NumberedEquation9.gif) |
(20) |
(Hardy and Wright 1979, p. 357).
The first few numbers 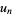 which are products of an odd number of distinct prime factors (Hardy 1999, p. 64; Ramanujan 2000, pp. xxiv and 21) are 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 30, 31, 37, 41, 42, 43, 47, ... (OEIS A030059).
which are products of an odd number of distinct prime factors (Hardy 1999, p. 64; Ramanujan 2000, pp. xxiv and 21) are 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 30, 31, 37, 41, 42, 43, 47, ... (OEIS A030059). 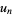 satisfies
satisfies
![sum_(n=1)^infty1/(u_n^s)=1/2[(zeta(s))/(zeta(2s))-zeta(s)]](https://mathworld.wolfram.com/images/equations/DistinctPrimeFactors/NumberedEquation10.gif) |
(21) |
(Hardy 1999, pp. 64-65). In addition, if 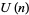 is the number of
is the number of 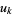 with
with 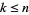 , then
, then
 |
(22) |
(Hardy 1999, pp. 64-65).
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 844, 1972.
Diaconis, P. "Asymptotic Expansions for the Mean and Variance of the Number of Prime Factors of a Number 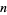 ." Dept. Statistics Tech. Report 96, Stanford, CA: Stanford University, 1976.
." Dept. Statistics Tech. Report 96, Stanford, CA: Stanford University, 1976.
Diaconis, P. "G. H. Hardy and Probability???" Bull. London Math. Soc. 34, 385-402, 2002.
Finch, S. "Two Asymptotic Series." December 10, 2003. https://algo.inria.fr/bsolve/.
Hardy, G. H. Ramanujan: Twelve Lectures on Subjects Suggested by His Life and Work, 3rd ed. New York: Chelsea, 1999.
Hardy, G. H. and Ramanujan, S. "The Normal Number of Prime Factors of a Number 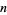 ." Quart. J. Math. 48, 76-92, 1917.
." Quart. J. Math. 48, 76-92, 1917.
Hardy, G. H. and Wright, E. M. "The Number of Prime Factors of 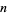 " and "The Normal Order of
" and "The Normal Order of 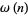 and
and 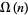 ." §22.10 and 22.11 in An Introduction to the Theory of Numbers, 5th ed. Oxford, England: Clarendon Press, pp. 354-358, 1979.
." §22.10 and 22.11 in An Introduction to the Theory of Numbers, 5th ed. Oxford, England: Clarendon Press, pp. 354-358, 1979.
Kac, M. Statistical Independence in Probability, Analysis and Number Theory. Washington, DC: Math. Assoc. Amer., p. 64, 1959.
Knuth, D. E. Selected Papers on Analysis of Algorithms. Stanford, CA: CSLI Publications, pp. 338-339, 2000.
Knuth, D. E. "Asymptotics for 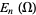 and
and  ." Cited by Finch (2003). Unpublished note, 2003.
." Cited by Finch (2003). Unpublished note, 2003.
Ramanujan, S. Collected Papers of Srinivasa Ramanujan (Ed. G. H. Hardy, P. V. S. Aiyar, and B. M. Wilson). Providence, RI: Amer. Math. Soc., 2000.
Sloane, N. J. A. Sequences A001221/M0056, A013939, A027748, A085548, and A091588 in "The On-Line Encyclopedia of Integer Sequences."
Sloane, N. J. A. and Plouffe, S. The Encyclopedia of Integer Sequences. San Diego, CA: Academic Press, 1995.
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















