

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Grothendieck,s Constant
المؤلف:
Finch, S. R.
المصدر:
"Grothendieck,s Constants." §3.11 in Mathematical Constants. Cambridge, England: Cambridge University Press
الجزء والصفحة:
...
26-2-2020
776
Let 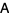 be an
be an  real square matrix with
real square matrix with  such that
such that
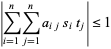 |
(1) |
for all real numbers 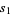 ,
, 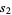 , ...,
, ..., 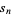 and
and 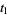 ,
, 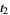 , ...,
, ..., 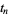 such that
such that 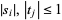 . Then Grothendieck showed that there exists a constant
. Then Grothendieck showed that there exists a constant 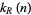 satisfying
satisfying
 |
(2) |
for all vectors 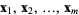 and
and 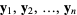 in a Hilbert space with norms
in a Hilbert space with norms 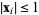 and
and 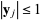 . The Grothendieck constant is the smallest possible value of
. The Grothendieck constant is the smallest possible value of 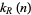 . For example, the best values known for small
. For example, the best values known for small  are
are
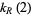 |
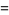 |
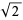 |
(3) |
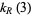 |
 |
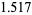 |
(4) |
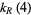 |
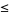 |
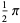 |
(5) |
(Krivine 1977, 1979; König 1992; Finch 2003, p. 236).
Now consider the limit
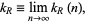 |
(6) |
which is related to Khinchin's constant and sometimes also denoted 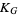 . Krivine (1977) showed that
. Krivine (1977) showed that
 |
(7) |
and postulated that
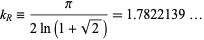 |
(8) |
(OEIS A088367). The conjecture was refuted in 2011 by Yury Makarychev, Mark Braverman, Konstantin Makarychev, and Assaf Naor, who showed that 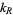 is strictly less than Krivine's bound (Makarychev 2011).
is strictly less than Krivine's bound (Makarychev 2011).
Similarly, if the numbers 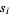 and
and 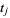 and matrix
and matrix 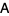 are taken as complex, then a similar set of constants
are taken as complex, then a similar set of constants 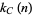 may be defined. These are known to satisfy
may be defined. These are known to satisfy
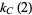 |
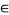 |
![[1.1526,1.2157]](http://mathworld.wolfram.com/images/equations/GrothendiecksConstant/Inline35.gif) |
(9) |
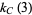 |
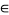 |
![[1.2108,1.2744]](http://mathworld.wolfram.com/images/equations/GrothendiecksConstant/Inline38.gif) |
(10) |
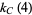 |
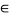 |
![[1.2413,1.3048]](http://mathworld.wolfram.com/images/equations/GrothendiecksConstant/Inline41.gif) |
(11) |
(Krivine 1977, 1979; König 1990, 1992; Finch 2003, p. 236).
The limit
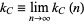 |
(12) |
satisfies
 |
(13) |
(Krivine 1977, 1979; Haagerup 1987; Finch 20003, p. 246), where the upper limit (OEIS A088374) is given by ![8/[pi(x_0+1)]](http://mathworld.wolfram.com/images/equations/GrothendiecksConstant/Inline42.gif) with
with
 |
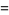 |
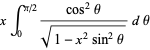 |
(14) |
 |
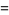 |
![1/x[E(x)-(1-x^2)K(x)],](http://mathworld.wolfram.com/images/equations/GrothendiecksConstant/Inline48.gif) |
(15) |
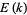 a complete elliptic integral of the second kind,
a complete elliptic integral of the second kind, 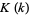 a complete elliptic integral of the first kind, and
a complete elliptic integral of the first kind, and  (OEIS A088373) the root of
(OEIS A088373) the root of
 |
(16) |
However, Haagerup (1987) has suggested that the upper limit (and presumable actual value) is incorrect and would more plausibly be given by
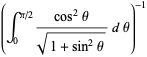 |
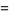 |
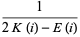 |
(17) |
 |
 |
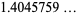 |
(18) |
(OEIS A088375; Finch 2003, pp. 236-237).
REFERENCES:
Finch, S. R. "Grothendieck's Constants." §3.11 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 235-237, 2003.
Fishburn, P. C. and Reeds, J. A. "Bell Inequalities, Grothendieck's Constant, and Root Two." SIAM J. Discr. Math. 7, 48-56, 1994.
Haagerup, U. "A New Upper Bound for the Complex Grothendieck Constant." Israeli J. Math. 60, 199-224, 1987.
König, H. "On the Complex Grothendieck Constant in the 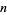 -Dimensional Case." In Geometry of Banach Spaces: Proceedings of the Conference Held in Linz, 1989 (Ed. P. F. X. Müller and W. Schachermauer). Cambridge, England: Cambridge University Press, pp. 181-198, 1990.
-Dimensional Case." In Geometry of Banach Spaces: Proceedings of the Conference Held in Linz, 1989 (Ed. P. F. X. Müller and W. Schachermauer). Cambridge, England: Cambridge University Press, pp. 181-198, 1990.
König, H. "Some Remarks on the Grothendieck Inequality." General Inequalities 6, Proc. 1990 Oberwolfach Conference (Ed. W. Walter). Basel, Switzerland: Birkhäuser, pp. 201-206, 1992.
Krivine, J.-L. "Sur la constante de Grothendieck." C. R. A. S. 284, 445-446, 1977.
Krivine, J.-L. "Constantes de Grothendieck et fonctions de type positif sur les spheres." Adv. Math. 31, 16-30, 1979.
Jameson, G. L. O. Summing and Nuclear Norms in Banach Space Theory. Cambridge, England: Cambridge University Press, 1987.
Le Lionnais, F. Les nombres remarquables. Paris: Hermann, p. 42, 1983.
Lindenstrauss, J. and Pełczyński, A. "Absolutely Summing Operators in 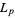 Spaces and Their Applications." Studia Math. 29, 275-326, 1968.
Spaces and Their Applications." Studia Math. 29, 275-326, 1968.
Makarychev, Y. "The Grothendieck Constant Is Strictly Smaller Than Krivine." Seminar. Cambridge, MA: MIT Computer Science and Artificial Intelligence Laboratory. Nov. 8, 2011.
Sloane, N. J. A. Sequences A088367, A088373, A088374, and A088375 in "The On-Line Encyclopedia of Integer Sequences."