


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 11-10-2021
Date: 18-11-2021
Date: 22-9-2021
|
An attracting set that has zero measure in the embedding phase space and has fractal dimension. Trajectories within a strange attractor appear to skip around randomly.
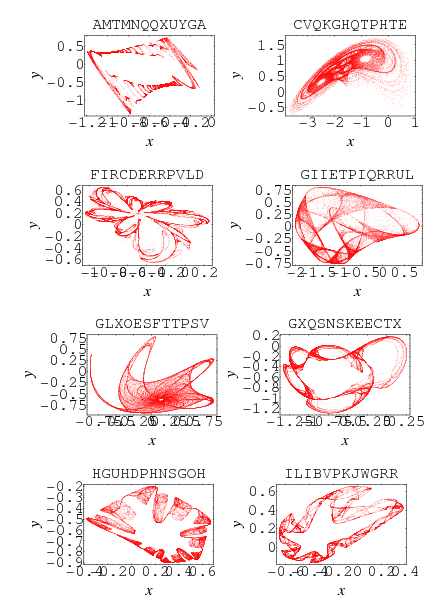

A selection of strange attractors for a general quadratic map
 |
 |
 |
(1) |
 |
 |
 |
(2) |
are illustrated above, where the letters  to
to  stand for coefficients of the quadratic from
stand for coefficients of the quadratic from  to 1.2 in steps of 0.1 (Sprott 1993c). These represent a small selection of the approximately 1.6% of all possible
to 1.2 in steps of 0.1 (Sprott 1993c). These represent a small selection of the approximately 1.6% of all possible  such maps that are chaotic (Sprott 1993bc).
such maps that are chaotic (Sprott 1993bc).
REFERENCES:
Benmizrachi, A.; Procaccia, I.; and Grassberger, P. "Characterization of Experimental (Noisy) Strange Attractors." Phys. Rev. A 29, 975-977, 1984.
Dewdney, A. K. "Probing the Strange Attractors of Chaos." Sci. Amer. 235, 90-93, 1976.
Farmer, J. D.; Ott, E.; and Yorke, J. A. "The Dimension of Chaotic Attractors." Physica 7D, 153, 1983.
Gleick, J. "Strange Attractors." Chaos: Making a New Science. New York: Penguin Books, pp. 119-153, 1988.
Grassberger, P. "On the Hausdorff Dimension of Fractal Attractors." J. Stat. Phys. 26, 173-179, 1981.
Grassberger, P. and Procaccia, I. "Measuring the Strangeness of Strange Attractors." Physica D 9, 189-208, 1983a.
Grassberger, P. and Procaccia, I. "Characterization of Strange Attractors." Phys. Rev. Let. 50, 346-349, 1983b.
Lauwerier, H. Fractals: Endlessly Repeated Geometric Figures. Princeton, NJ: Princeton University Press, pp. 137-138, 1991.
Peitgen, H.-O. and Richter, D. H. The Beauty of Fractals: Images of Complex Dynamical Systems. New York: Springer-Verlag, 1986.
Pickover, C. "A Note on Rendering 3-D Strange-Attractors." Comput. & Graphics 12, 263, 1988.
Sprott, J. C. Strange Attractors: Creating Patterns in Chaos. New York: Henry Holt, 1993a.
Sprott, J. C. "How Common Is Chaos?" Phys. Lett. A 173, 21, 1993b.
Sprott, J. C. "Automatic Generation of Strange Attractors." Comput. & Graphics 17, 325-332, 1993c. Reprinted in Chaos and Fractals, A Computer Graphical Journey: Ten Year Compilation of Advanced Research (Ed. C. A. Pickover). Amsterdam, Netherlands: Elsevier, pp. 53-60, 1998.
Viana, M. "What's New on Lorenz Strange Attractors." Math. Intell. 22, 6-19.



|
|
|
|
التوتر والسرطان.. علماء يحذرون من "صلة خطيرة"
|
|
|
|
|
|
|
مرآة السيارة: مدى دقة عكسها للصورة الصحيحة
|
|
|
|
|
|
|
نحو شراكة وطنية متكاملة.. الأمين العام للعتبة الحسينية يبحث مع وكيل وزارة الخارجية آفاق التعاون المؤسسي
|
|
|