k-Tuple Conjecture

The first of the Hardy-Littlewood conjectures. The -tuple conjecture states that the asymptotic number of prime constellations can be computed explicitly. In particular, unless there is a trivial divisibility condition that stops 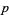, , 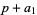...,  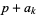from consisting of primes infinitely often, then such prime constellations will occur with an asymptotic density which is computable in terms of 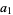, ..., 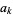. Let 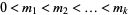, then the 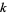-tuple conjecture predicts that the number of primes  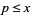such that 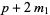, , 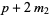...,  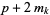are all prime is

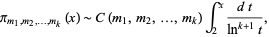(1)

where

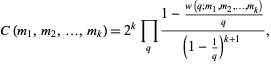(2)

the product is over odd primes 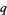, and

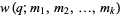(3)

denotes the number of distinct residues of 0, , ...,  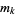(mod 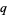) (Halberstam and Richert 1974, Odlyzko et al. 1999). If 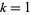, then this becomes

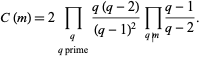(4)

This conjecture is generally believed to be true, but has not been proven (Odlyzko et al. 1999).

The twin prime conjecture

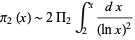(5)

is a special case of the 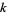-tuple conjecture with , where 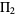 is known as the twin primes constant.

The following special case of the conjecture is sometimes known as the prime patterns conjecture. Let 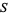 be a finite set of integers. Then it is conjectured that there exist infinitely many  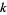for which  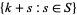are all prime iff  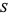does not include all the residues of any prime. This conjecture also implies that there are arbitrarily long arithmetic progressions of primes.

REFERENCES:

Brent, R. P. "The Distribution of Small Gaps Between Successive Primes." Math. Comput. 28, 315-324, 1974.

Brent, R. P. "Irregularities in the Distribution of Primes and Twin Primes." Math. Comput. 29, 43-56, 1975.

Halberstam, E. and Richert, H.-E. Sieve Methods. New York: Academic Press, 1974.

Hardy, G. H. and Littlewood, J. E. "Some Problems of 'Partitio Numerorum.' III. On the Expression of a Number as a Sum of Primes." Acta Math. 44, 1-70, 1923.

Odlyzko, A.; Rubinstein, M.; and Wolf, M. "Jumping Champions." Experiment. Math. 8, 107-118, 1999.

Riesel, H. Prime Numbers and Computer Methods for Factorization, 2nd ed. Boston, MA: Birkhäuser, pp. 66-68, 1994.