


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 15-10-2019
Date: 29-9-2019
Date: 20-8-2018
|
The spherical harmonics can be generalized to vector spherical harmonics by looking for a scalar function 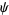 and a constant vector
and a constant vector 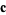 such that
such that
 |
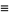 |
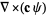 |
(1) |
 |
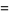 |
 |
(2) |
 |
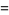 |
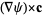 |
(3) |
 |
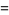 |
 |
(4) |
so
 |
(5) |
Now interchange the order of differentiation and use the fact that multiplicative constants can be moving inside and outside the derivatives to obtain
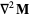 |
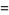 |
 |
(6) |
 |
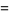 |
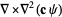 |
(7) |
 |
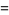 |
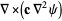 |
(8) |
and
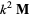 |
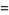 |
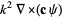 |
(9) |
 |
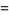 |
 |
(10) |
Putting these together gives
![del ^2M+k^2M=del x[c(del ^2psi+k^2psi)],](http://mathworld.wolfram.com/images/equations/VectorSphericalHarmonic/NumberedEquation2.gif) |
(11) |
so 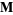 satisfies the vector Helmholtz differential equation if
satisfies the vector Helmholtz differential equation if 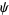 satisfies the scalar Helmholtz differential equation
satisfies the scalar Helmholtz differential equation
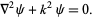 |
(12) |
Construct another vector function
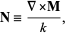 |
(13) |
which also satisfies the vector Helmholtz differential equation since
 |
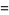 |
 |
(14) |
 |
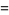 |
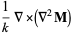 |
(15) |
 |
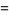 |
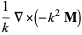 |
(16) |
 |
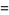 |
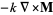 |
(17) |
 |
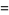 |
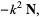 |
(18) |
which gives
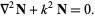 |
(19) |
We have the additional identity
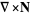 |
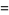 |
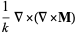 |
(20) |
 |
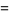 |
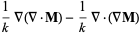 |
(21) |
 |
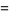 |
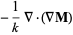 |
(22) |
 |
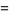 |
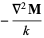 |
(23) |
 |
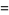 |
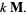 |
(24) |
In this formalism, 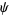 is called the generating function and
is called the generating function and  is called the pilot vector. The choice of generating function is determined by the symmetry of the scalar equation, i.e., it is chosen to solve the desired scalar differential equation. If
is called the pilot vector. The choice of generating function is determined by the symmetry of the scalar equation, i.e., it is chosen to solve the desired scalar differential equation. If 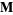 is taken as
is taken as
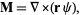 |
(25) |
where  is the radius vector, then
is the radius vector, then 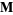 is a solution to the vector wave equation in spherical coordinates. If we want vector solutions which are tangential to the radius vector,
is a solution to the vector wave equation in spherical coordinates. If we want vector solutions which are tangential to the radius vector,
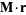 |
 |
 |
(26) |
 |
 |
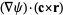 |
(27) |
 |
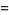 |
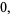 |
(28) |
so
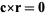 |
(29) |
and we may take
 |
(30) |
(Arfken 1985, pp. 707-711; Bohren and Huffman 1983, p. 88).
A number of conventions are in use. Hill (1954) defines
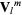 |
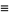 |
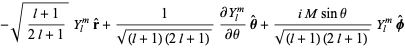 |
(31) |
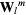 |
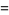 |
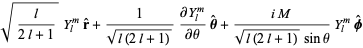 |
(32) |
 |
 |
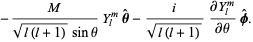 |
(33) |
Morse and Feshbach (1953) define vector harmonics called 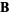 ,
, 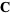 , and
, and 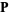 using rather complicated expressions.
using rather complicated expressions.
REFERENCES:
Arfken, G. "Vector Spherical Harmonics." §12.11 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 707-711, 1985.
Blatt, J. M. and Weisskopf, V. "Vector Spherical Harmonics." Appendix B, §1 in Theoretical Nuclear Physics. New York: Wiley, pp. 796-799, 1952.
Bohren, C. F. and Huffman, D. R. Absorption and Scattering of Light by Small Particles. New York: Wiley, 1983.
Hill, E. H. "The Theory of Vector Spherical Harmonics." Amer. J. Phys. 22, 211-214, 1954.
Jackson, J. D. Classical Electrodynamics, 2nd ed. New York: Wiley, pp. 744-755, 1975.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part II. New York: McGraw-Hill, pp. 1898-1901, 1953.



|
|
|
|
التوتر والسرطان.. علماء يحذرون من "صلة خطيرة"
|
|
|
|
|
|
|
مرآة السيارة: مدى دقة عكسها للصورة الصحيحة
|
|
|
|
|
|
|
نحو شراكة وطنية متكاملة.. الأمين العام للعتبة الحسينية يبحث مع وكيل وزارة الخارجية آفاق التعاون المؤسسي
|
|
|