Weierstrass Sigma Function

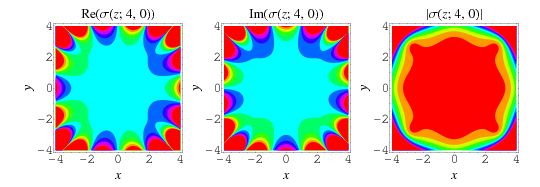
The quasiperiodic function defined by

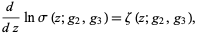(1)

where 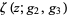 is the Weierstrass zeta function and

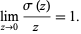(2)

(As in the case of other Weierstrass elliptic functions, the invariants 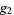 and  are frequently suppressed for compactness.) Then

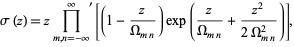(3)

where the term with 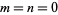 is omitted from the product and .

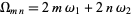Amazingly, 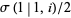, where  is the Weierstrass sigma function with half-periods  and 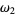, has a closed form in terms of 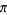, , 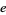and 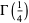. This constant is known as the Weierstrass constant.

In addition,  satisfies

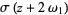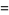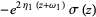			(4)
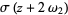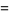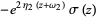			(5)

and

(6)

for 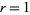, 2, 3. The function is implemented in the Wolfram Language as WeierstrassSigma[u, g2, g3].

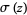 can be expressed in terms of Jacobi theta functions using the expression

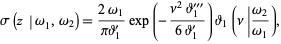(7)

where 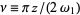, and

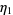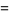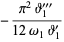			(8)
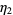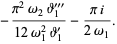			(9)

There is a beautiful series expansion for , given by the double series

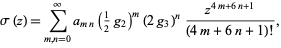(10)

where 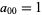,  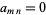for either subscript negative, and other values are gives by the recurrence relation

(11)

(Abramowitz and Stegun 1972, pp. 635-636). The following table gives the values of the  coefficients for small and .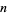

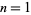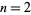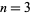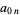	1	-3	-54	14904
	-1	-18	4968	502200
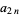	-9	513	257580	162100440
	69	33588	20019960	-9465715080
	321	2808945	-376375410	-4582619446320
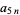	160839	-41843142	-210469286736	-1028311276281264

REFERENCES:

Abramowitz, M. and Stegun, I. A. (Eds.). "Weierstrass Elliptic and Related Functions." Ch. 18 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 627-671, 1972.

Brezhnev, Y. V. "Uniformisation: On the Burnside Curve 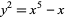." 9 Dec 2001. http://arxiv.org/abs/math.CA/0111150.

Knopp, K. "Example: Weierstrass's -Function." §2d in Theory of Functions Parts I and II, Two Volumes Bound as One, Part II.New York: Dover, pp. 27-30, 1996.

Tölke, F. "Spezielle Weierstraßsche Sigma-Funktionen." Ch. 9 in Praktische Funktionenlehre, dritter Band: Jacobische elliptische Funktionen, Legendresche elliptische Normalintegrale und spezielle Weierstraßsche Zeta- und Sigma Funktionen. Berlin: Springer-Verlag, pp. 164-180, 1967.

Whittaker, E. T. and Watson, G. N. "The Function 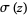." §20.42 in A Course in Modern Analysis, 4th ed. Cambridge, England: Cambridge University Press, pp. 447-448, 450-452, and 458-461, 1990.